Übungen3: Unterschied zwischen den Versionen
| Zeile 26: | Zeile 26: | ||
|} | |} | ||
| + | |||
| + | === Übung 2: Lückentext === | ||
| + | |||
| + | <div class="lueckentext-quiz"> | ||
| + | Die Graph der Funktion f mit f(x)=ax²+bx heißt <strong> Parabel </strong>. Ist a = 1, so heißt der Graph <strong> Normalparabel</strong>.<br> | ||
| + | Quadratische Funktionen liegen <strong>symmetrisch </strong> zur <strong>y-Achse</strong>.<br> | ||
| + | Der Punkt S (0;0) heißt <strong>Scheitel </strong>.<br> | ||
| + | Für a>0 gilt: Je <strong>größer </strong> a ist, desto steiler ist die Parabel. <br> | ||
| + | Für a>0 gilt: Je kleiner a ist, desto <strong> weiter </strong> ist die Parabel. <br> | ||
| + | </div> | ||
| + | <br> | ||
| + | <br> | ||
| + | <br> | ||
| + | <br> | ||
| + | |||
| + | |||
| + | ===Übung 3: Term und Graph zuordnen === | ||
| + | <div class="lueckentext-quiz"> | ||
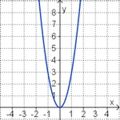
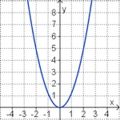
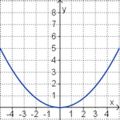
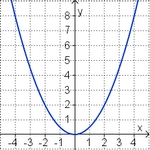
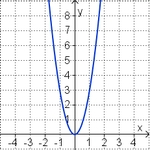
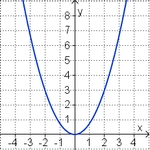
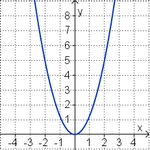
| + | Ordne den Funktionsgraphen den richtigen Term zu. | ||
| + | |||
| + | {| | ||
| + | |- | ||
| + | | [[Bild:Parabel_a_0_5a.jpg|150px]] || [[Bild:Parabel_a_2a.jpg|150px]] || [[Bild:Parabel_a_3a.jpg|150px]] || [[Bild:Parabel_a_0_75a.jpg|150px]] || [[Bild:Parabel_a_1_25a.jpg|150px]] || [[Bild:Parabel_a_0_2a.jpg|150px]] | ||
| + | |- | ||
| + | | <strong> 0,5x<sup>2</sup> </strong> || <strong> 2x<sup>2</sup> </strong> || <strong> 3x<sup>2</sup> </strong> || <strong> 0,75x<sup>2</sup> </strong> || <strong> 1,25x<sup>2</sup> </strong> || <strong> 0,2x<sup>2</sup> </strong> | ||
| + | |} | ||
| + | |||
| + | </div> | ||
| + | <br><br><br><br> | ||
| + | |||
| + | |||
| + | === Übung 4: Multiple Choice === | ||
| + | <div class="multiplechoice-quiz"> | ||
| + | |||
| + | |||
| + | Kreuze jeweils '''alle''' richtigen Aussagen an. | ||
| + | |||
| + | <big>f(x) = 3,5x<sup>2</sup></big> (!Die Parabel ist nach unten geöffnet.) (Die Parabel ist nach oben geöffnet.) (Die Parabel ist enger als die Normalparabel.) (!Die Parabel ist weiter als die Normalparabel.) (Der Punkt [2|14] liegt auf dem Graphen.) (Der Punkt [14|2] liegt nicht auf dem Graphen.) | ||
| + | |||
| + | <big>f(x) = - 0,5x<sup>2</sup></big> (Die Parabel ist nach unten geöffnet.) (!Die Parabel ist nach oben geöffnet.) (!Die Parabel ist enger als die Normalparabel.) (Die Parabel ist weiter als die Normalparabel.) (Der Punkt [2|-2] liegt auf dem Graphen.) (!Der Punkt [2|2] liegt auf dem Graphen.) | ||
| + | |||
| + | <big>f(x) = - 2x<sup>2</sup></big> (Die Parabel ist nach unten geöffnet.) (!Die Parabel ist nach oben geöffnet.) (Die Parabel ist enger als die Normalparabel.) (!Die Parabel ist weiter als die Normalparabel.) (!Der Punkt [0|-2] liegt auf dem Graphen.) (Der Punkt [1|2] liegt oberhalb des Graphen.) | ||
| + | |||
| + | <big>f(x) = 0,2x<sup>2</sup></big> (!Die Parabel ist nach unten geöffnet.) (Die Parabel ist nach oben geöffnet.) (!Die Parabel ist enger als die Normalparabel.) (Die Parabel ist weiter als die Normalparabel.) (!Der Punkt [-1|2] liegt auf dem Graphen.) (Der Punkt [-1|1] liegt oberhalb des Graphen.) | ||
| + | </div> | ||
Version vom 27. Februar 2009, 23:25 Uhr
Quadratische Funktionen/Übungen1 - Quadratische Funktionen/Übungen2 - Quadratische Funktionen/Übungen3 - Quadratische Funktionen/Abschlusstest - - Quadratische Funktionen/Rest
Inhaltsverzeichnis |
Memory
|
Übung 2: Lückentext
Die Graph der Funktion f mit f(x)=ax²+bx heißt Parabel . Ist a = 1, so heißt der Graph Normalparabel.
Quadratische Funktionen liegen symmetrisch zur y-Achse.
Der Punkt S (0;0) heißt Scheitel .
Für a>0 gilt: Je größer a ist, desto steiler ist die Parabel.
Für a>0 gilt: Je kleiner a ist, desto weiter ist die Parabel.
Übung 3: Term und Graph zuordnen
Ordne den Funktionsgraphen den richtigen Term zu.
 |
 |
 |
 |
 |
|
| 0,5x2 | 2x2 | 3x2 | 0,75x2 | 1,25x2 | 0,2x2 |
Übung 4: Multiple Choice
Kreuze jeweils alle richtigen Aussagen an.
f(x) = 3,5x2 (!Die Parabel ist nach unten geöffnet.) (Die Parabel ist nach oben geöffnet.) (Die Parabel ist enger als die Normalparabel.) (!Die Parabel ist weiter als die Normalparabel.) (Der Punkt [2|14] liegt auf dem Graphen.) (Der Punkt [14|2] liegt nicht auf dem Graphen.)
f(x) = - 0,5x2 (Die Parabel ist nach unten geöffnet.) (!Die Parabel ist nach oben geöffnet.) (!Die Parabel ist enger als die Normalparabel.) (Die Parabel ist weiter als die Normalparabel.) (Der Punkt [2|-2] liegt auf dem Graphen.) (!Der Punkt [2|2] liegt auf dem Graphen.)
f(x) = - 2x2 (Die Parabel ist nach unten geöffnet.) (!Die Parabel ist nach oben geöffnet.) (Die Parabel ist enger als die Normalparabel.) (!Die Parabel ist weiter als die Normalparabel.) (!Der Punkt [0|-2] liegt auf dem Graphen.) (Der Punkt [1|2] liegt oberhalb des Graphen.)
f(x) = 0,2x2 (!Die Parabel ist nach unten geöffnet.) (Die Parabel ist nach oben geöffnet.) (!Die Parabel ist enger als die Normalparabel.) (Die Parabel ist weiter als die Normalparabel.) (!Der Punkt [-1|2] liegt auf dem Graphen.) (Der Punkt [-1|1] liegt oberhalb des Graphen.)
