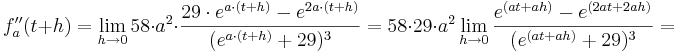Lösung b): Unterschied zwischen den Versionen

Aus RMG-Wiki
Wechseln zu: Navigation, Suche
K (→‎Beweis für Wendepunkt:)
(→‎Suche nach dem Wendepunkt:)
Zeile 30: Zeile 30:
 
  <math>f''_{a}(t) = 58\cdot a^{2} \cdot \frac {29\cdot e^{at} - e^{2at}}{(e^{at}+29)^{3}} = 0 </math>
 
  <math>f''_{a}(t) = 58\cdot a^{2} \cdot \frac {29\cdot e^{at} - e^{2at}}{(e^{at}+29)^{3}} = 0 </math>
 
   
 
   
−
  <math>58\cdot a^{2} (29\cdot e^{at} - e^{2at}) = 0</math>   <math>| : 58\cdot a^{2} \Rightarrow (a \neq 0)</math>
+
  <math>58\cdot a^{2} (29\cdot e^{at} - e^{2at}) = 0</math>                             <math>| : 58\cdot a^{2} \Rightarrow (a \neq 0)</math>
−
<math>(29\cdot e^{at} - e^{2at}) = 0</math>         | + e^{2at}
+
        <math>(29\cdot e^{at} - e^{2at}) = 0</math>                             <math>| + e^{2at}\;</math>  
−
  <math>29 \cdot e^{at} = e^{2at}</math>               | ln
+
                <math>29 \cdot e^{at} = e^{2at}</math>                           <math>| ln\;</math>
−
<math>ln(29\cdot e^{at}) = ln(e^{2at})</math>
+
            <math>ln(29\cdot e^{at}) = ln(e^{2at})</math>
−
ln(29) + ln(e^{at}) = ln(e^{2at})           | - ln(e^{at})
+
      <math>ln(29) + ln(e^{at}) = ln(e^{2at})\;</math>                      <math>| - ln(e^{at})\;</math>
−
ln(29) = ln(e^{2at}) - ln(e^{at})
+
                <math>ln(29) = ln(e^{2at}) - ln(e^{at})\;</math>
−
<math>ln(29) = 2\cdot a\cdot t \cdot ln(e) - a\cdot t\cdot ln(e)</math>    (ln(e)=1)
+
                <math>ln(29) = 2\cdot a\cdot t \cdot ln(e) - a\cdot t\cdot ln(e)</math>    (ln(e)=1)
−
<math>ln(29) = 2\cdot a\cdot t - a\cdot t</math>
+
                <math>ln(29) = 2\cdot a\cdot t - a\cdot t</math>
−
<math>ln(29) = a\cdot t</math>
+
                <math>ln(29) = a\cdot t</math>
−
<math>t = \frac {ln29} {a}</math>
+
                      <math>t = \frac {ln29} {a}</math>
  
 
===<u>Beweis für Wendepunkt:</u>===
 
===<u>Beweis für Wendepunkt:</u>===

Version vom 16. Januar 2010, 10:16 Uhr

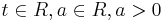
y = f_{a}(t) = \frac{2\cdot e^{at}}{e^{at}+29}, t\in R, a\in R, a>0

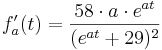
f'_{a} (t) = \frac{58\cdot a\cdot e^{at} }{(e^{at}+29) ^{2}}

Inhaltsverzeichnis

Untersuchen sie die Funktionen fa auf Nullstellen und lokale Extremstellen

Suche nach Nullstellen:

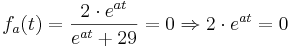
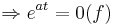
f_{a}(t) = \frac{2\cdot e^{at}}{e^{at}+29} = 0 \Rightarrow 2\cdot e^{at} = 0 \Rightarrow e^{at} = 0 (f)

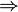\Rightarrow keine Nullstellen, da die e-Fkt. nie 0 wird und somit der Ausdruck 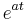e^{at} ebenfalls nie 0 werden kann

Suche nach Extremstellen:

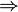f'_{a} (t) = \frac{58\cdot a\cdot e^{at} }{(e^{at}+29) ^{2}} = 0 \Rightarrow 58\cdot a \cdot e^{at} = 0 \Rightarrow e^{at} = 0 (f)

\Rightarrow keine Extremstellen, da die e-Fkt. nie 0 wird und somit der Ausdruck 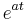e^{at} ebenfalls nie 0 werden kann


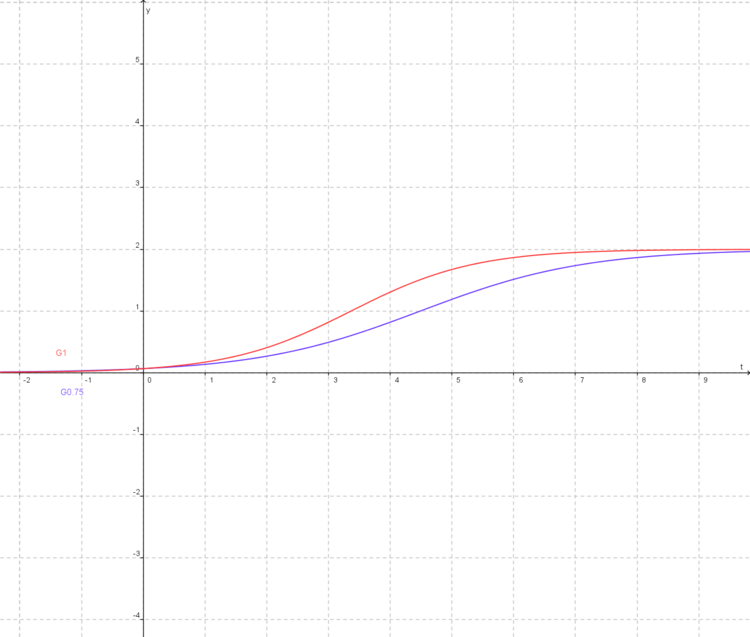
Jeder Graph Ga bestitzt genau einen Wendepunkt Wa. Zeigen sie, dass die Wendepunkte Wa auf einer parallelen zur t-Achse liegen

Die 2. Ableitung:

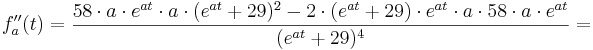
f''_{a}(t) = \frac{58\cdot a \cdot e^{at}\cdot a\cdot(e^{at}+29)^{2} - 2 \cdot(e^{at} + 29)\cdot e^{at}\cdot a \cdot 58 \cdot a \cdot e^{at} }{(e^{at} + 29) ^{4} } =
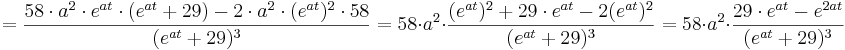
= \frac{58\cdot a^{2} \cdot e^{at}\cdot (e^{at} + 29) - 2\cdot a^{2} \cdot (e^{at})^{2}\cdot 58 }{(e^{at}+29)^{3}} = 58\cdot a^{2}\cdot \frac{(e^{at})^{2} + 29\cdot e^{at} - 2(e^{at})^2}{(e^{at} + 29)^{3}} = 58\cdot a^{2} \cdot \frac {29\cdot e^{at} - e^{2at}}{(e^{at}+29)^{3}}

Suche nach dem Wendepunkt:

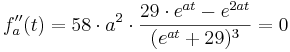
f''_{a}(t) = 58\cdot a^{2} \cdot \frac {29\cdot e^{at} - e^{2at}}{(e^{at}+29)^{3}} = 0 

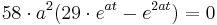
58\cdot a^{2} (29\cdot e^{at} - e^{2at}) = 0                              | : 58\cdot a^{2} \Rightarrow (a \neq 0)
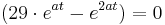
       (29\cdot e^{at} - e^{2at}) = 0                              | + e^{2at}\;  
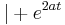
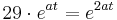
               29 \cdot e^{at} = e^{2at}                            | ln\;
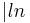
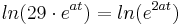
           ln(29\cdot e^{at}) = ln(e^{2at})
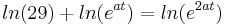
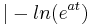
      ln(29) + ln(e^{at}) = ln(e^{2at})\;                       | - ln(e^{at})\;
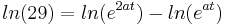
                ln(29) = ln(e^{2at}) - ln(e^{at})\;
                ln(29) = 2\cdot a\cdot t \cdot ln(e) - a\cdot t\cdot ln(e)    (ln(e)=1)
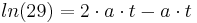
                ln(29) = 2\cdot a\cdot t - a\cdot t
                ln(29) = a\cdot t
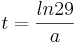
                      t = \frac {ln29} {a}

Beweis für Wendepunkt:

1. Möglichkeit: Die H-Methode

Man nähert sich dem möglichen Wendepunkt mit Hilfe eines Grenzwertes an und versucht herauszufinden, ob ein Vorzeichenwechsel am Wendepunkt stattfindet. Falls es einen Vorzeichenwechsel geben sollte, ist dies der eindeutige Beweis für einen Wendepunkt an dieser Stelle. 

f''_{a}(t+h) = \lim_{h \to 0} 58\cdot a^{2}\cdot \frac {29\cdot e^{a\cdot(t+h)} - e^{2a\cdot(t+h)}}{(e^{a\cdot (t+h)}+29)^{3}} = 58\cdot 29\cdot a^{2}\lim_{h \to 0} \frac {e^{(at + ah)} - e^{(2at + 2ah)}}{(e^{(at + ah)} + 29)^{3}} =

2. Möglichkeit:

3. Möglichkeit:

Zeichnen sie die Graphen G0,75 und G1 in ein und dasselbe Koordinatensystem und schlussfolgern Sie, welchen Einfluss der Parameter a auf den Verlauf der Graphen Ga hat

Der Graph

Graph-facharbeit1.png

Von „http://rmg.zum.de/index.php?title=Facharbeit_Mathematik_Straßheimer/Die_Aufgabe/Lösung_b)&oldid=26913“