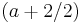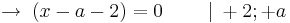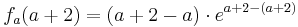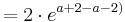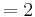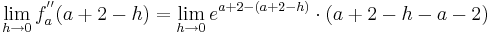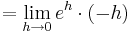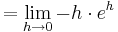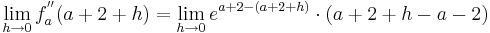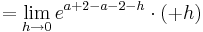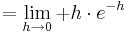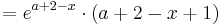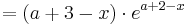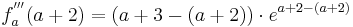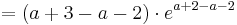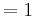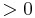Lösung: Wendepunkte: Unterschied zwischen den Versionen

Aus RMG-Wiki
Wechseln zu: Navigation, Suche
(→‎1. Möglichkeit; H-Methode)
 
(9 dazwischenliegende Versionen von einem Benutzer werden nicht angezeigt)
Zeile 7: Zeile 7:
 
<math>f_a^{''} (x) = e^{a+2-x}\cdot ( x - a - 2 )</math>
 
<math>f_a^{''} (x) = e^{a+2-x}\cdot ( x - a - 2 )</math>
  
−
Um mögl. Wendepunkte zu bestimmen benötigt man die zweite Ableitung.
+
Um mögliche Wendepunkte zu bestimmen benötigt man die zweite Ableitung.
  
−
Mögl. Wendepunkte tretten für <math>f_a^{''} (x) = 0\;</math> auf.<br />
+
Die zweite Ableitung einer Funktion beschreibt das Krümmungsverhalten dieser. Dieses ändert sich für <math>f_a^{''} (x) = 0\;</math> und deshalb könnte ein möglicher Wendepunkt auftreten.
  
  
Zeile 18: Zeile 18:
  
  
−
Möglicher Wendepunkt bei <math>x = a + 2 \; </math>
+
Möglicher Wendepunkt bei <math>x = a + 2 \; </math><br />
 +
Einsetzen des möglichen Wendepunkts in die Funktion.
  
  
Zeile 34: Zeile 35:
  
  
−
==== 1. Möglichkeit ====
+
==== 1. Möglichkeit; H-Methode ====
  
−
H-Methode , VZW des Krümmungsverhaltens
+
Wenn es einen Vorzeichenwechsel (VZW) des Krümmungsverhaltens der Funktion am möglichen Wendepunkt gibt, kann man von einem Wendepunkt sprechen.
  
 +
 +
'''I.<br />'''
 
: <math>\lim_{h\to 0}f_a^{''} ( a + 2  - h ) = \lim_{h\to 0}e^{a + 2 - (a + 2 - h )}\cdot ( a + 2 - h - a - 2 )</math><br />
 
: <math>\lim_{h\to 0}f_a^{''} ( a + 2  - h ) = \lim_{h\to 0}e^{a + 2 - (a + 2 - h )}\cdot ( a + 2 - h - a - 2 )</math><br />
−
::::::                                          <math>=\lim_{h\to 0} e^{a + 2 - a- 2 + h }\cdot (- h)</math><br />
+
:::::::                                          <math>=\lim_{h\to 0} e^{a + 2 - a- 2 + h }\cdot (- h)</math><br />
−
::::::                                          <math>= \lim_{h\to 0}e^{h }\cdot ( -h )</math><br />
+
:::::::                                          <math>= \lim_{h\to 0}e^{h }\cdot ( -h )</math><br />
−
::::::                                          <math>= \lim_{h\to 0}-h\cdot e^{h }</math><br />
+
:::::::                                          <math>= \lim_{h\to 0}-h\cdot e^{h }</math><br />
−
:: <math>\lim_{h\to 0}f_a^{''} ( a + 2 -  h )<0</math>
+
<math>\lim_{h\to 0}f_a^{''} ( a + 2 -  h )<0</math>
  
 +
<math>\rightarrow</math>  An der Stelle  <math>\lim_{h\to 0}f_a^{''}( a +2+ h )\;</math> ist der Graph linksgekrümmt.(I)
  
  
 +
'''II.'''<br />
 
: <math>\lim_{h\to 0}f_a^{''} ( a + 2 +  h ) =\lim_{h\to 0} e^{a + 2 - (a + 2 + h )}\cdot ( a + 2 + h - a - 2 )</math><br />
 
: <math>\lim_{h\to 0}f_a^{''} ( a + 2 +  h ) =\lim_{h\to 0} e^{a + 2 - (a + 2 + h )}\cdot ( a + 2 + h - a - 2 )</math><br />
−
::::::                                          <math>=\lim_{h\to 0} e^{a + 2 - a- 2 - h }\cdot (+ h)</math><br />
+
:::::::                                          <math>=\lim_{h\to 0} e^{a + 2 - a- 2 - h }\cdot (+ h)</math><br />
−
::::::                                          <math>= \lim_{h\to 0}e^{-h }\cdot ( +h )</math><br />
+
:::::::                                          <math>= \lim_{h\to 0}e^{-h }\cdot ( +h )</math><br />
−
::::::                                          <math>= \lim_{h\to 0}+h\cdot e^{-h }</math><br />
+
:::::::                                          <math>= \lim_{h\to 0}+h\cdot e^{-h }</math><br />
−
:: <math>\lim_{h\to 0}f_a^{''} ( a + 2 +  h )>0</math>
+
<math>\lim_{h\to 0}f_a^{''} ( a + 2 +  h )>0</math>
 
   
 
   
−
<math>\rightarrow</math>  VZW bei <math>x = a + 2\;</math><br />
+
<math>\rightarrow</math>  An der Stelle  <math>\lim_{h\to 0}f_a^{'}(a+2-h )\;</math> ist der Graph rechtsgekrümmt.(II)
−
<math>\rightarrow</math>  Wendepunkt bei <math>\;( a + 2 / 2 )</math>
+
 
 +
 
 +
Aus (I) und (II) folgt:<br />
 +
VZW bei <math>x = a + 2\;</math><br />
 +
<math>\Rightarrow</math>  Wendepunkt bei <math>\;( a + 2 / 2 )\;</math>  
  
  
−
<u>zur Verdeutlichung</u>
+
<u>zur Verdeutlichung:</u>
  
 
{| class="wikitable centersortable"
 
{| class="wikitable centersortable"
Zeile 106: Zeile 115:
 
<math>\rightarrow</math>  WP <math>( a + 2 / 2 )\;</math>
 
<math>\rightarrow</math>  WP <math>( a + 2 / 2 )\;</math>
  
−
==== 2. Möglichkeit ====
+
==== 2. Möglichkeit; 3.Ableitung ====
  
 
Verwendung der dritten Ableitung
 
Verwendung der dritten Ableitung
Zeile 130: Zeile 139:
 
::::                                      <math>= 1\cdot e^{0}</math><br />
 
::::                                      <math>= 1\cdot e^{0}</math><br />
 
::::                                      <math>= 1\;</math><br />  
 
::::                                      <math>= 1\;</math><br />  
−
::::                                      <math>> 0\;</math>
+
::::                                      <math>> 0\;</math><br />
 +
 
  
 
<math>\rightarrow</math>  WP <math>( a + 2 / 2 )\;</math>
 
<math>\rightarrow</math>  WP <math>( a + 2 / 2 )\;</math>

Aktuelle Version vom 26. Januar 2010, 19:43 Uhr

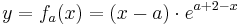y = f_a (x) = ( x - a )\cdot e^{a+2-x} mit x\in R ; a\in R

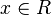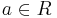
Inhaltsverzeichnis

Wendepunkte

Zweite Ableitung: siehe Überprüfung des Extrempunkts; 2. Möglichkeit

f_a^{''} (x) = e^{a+2-x}\cdot ( x - a - 2 )

Um mögliche Wendepunkte zu bestimmen benötigt man die zweite Ableitung.

Die zweite Ableitung einer Funktion beschreibt das Krümmungsverhalten dieser. Dieses ändert sich für 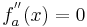f_a^{''} (x) = 0\; und deshalb könnte ein möglicher Wendepunkt auftreten.


f_a^{''} (x) = 0\;
e^{a+2-x}\cdot ( x - a - 2 ) = 0 \;\;\;\;\;\; | \; e^{a+2-x} > 0
\rightarrow \; ( x - a - 2 ) = 0 \;\;\;\;\;\;\;\; | \; + 2 ; + a
x = a + 2\;


Möglicher Wendepunkt bei x = a + 2 \;
Einsetzen des möglichen Wendepunkts in die Funktion.


f_a ( a + 2 ) = ( a + 2 - a )\cdot e^{a+2-(a + 2 )}
= 2\cdot e^{a+2-a - 2 )}
= 2\cdot e^0
= 2\;


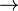\rightarrow mög. WP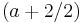 \; ( a + 2 / 2 )


Überprüfung des Wendepunkts

1. Möglichkeit; H-Methode

Wenn es einen Vorzeichenwechsel (VZW) des Krümmungsverhaltens der Funktion am möglichen Wendepunkt gibt, kann man von einem Wendepunkt sprechen.


I.

\lim_{h\to 0}f_a^{''} ( a + 2 - h ) = \lim_{h\to 0}e^{a + 2 - (a + 2 - h )}\cdot ( a + 2 - h - a - 2 )
=\lim_{h\to 0} e^{a + 2 - a- 2 + h }\cdot (- h)
= \lim_{h\to 0}e^{h }\cdot ( -h )
= \lim_{h\to 0}-h\cdot e^{h }

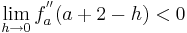
\lim_{h\to 0}f_a^{''} ( a + 2 - h )<0

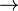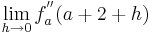\rightarrow An der Stelle \lim_{h\to 0}f_a^{''}( a +2+ h )\; ist der Graph linksgekrümmt.(I)


II.

\lim_{h\to 0}f_a^{''} ( a + 2 + h ) =\lim_{h\to 0} e^{a + 2 - (a + 2 + h )}\cdot ( a + 2 + h - a - 2 )
=\lim_{h\to 0} e^{a + 2 - a- 2 - h }\cdot (+ h)
= \lim_{h\to 0}e^{-h }\cdot ( +h )
= \lim_{h\to 0}+h\cdot e^{-h }

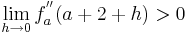
\lim_{h\to 0}f_a^{''} ( a + 2 + h )>0

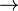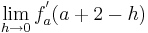\rightarrow An der Stelle \lim_{h\to 0}f_a^{'}(a+2-h )\; ist der Graph rechtsgekrümmt.(II)


Aus (I) und (II) folgt:
VZW bei x = a + 2\;
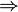\Rightarrow Wendepunkt bei \;( a + 2 / 2 )\;


zur Verdeutlichung:

Krümmungsverhalten
x<2+a x=2+a x>2+a
ea + 2 - x + +
( x - a - 2 ) - +
fa ( x ) - +

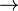\rightarrow WP ( a + 2 / 2 )\;

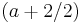
2. Möglichkeit; 3.Ableitung

Verwendung der dritten Ableitung

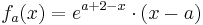
f_a (x) = e^{a+2-x}\cdot ( x - a)

f_a^{'} (x) = e^{a+2-x}\cdot ( 1+a-x )

f_a^{''} (x) = e^{a+2-x}\cdot ( x - a - 2 )


Um die dritte Ableitung zu bekommen muss man hier die Produktregel verwenden. [Hilfe zur Produktregel]

f_a^{'''} (x) = e^{a+2-x}\cdot ( x - a - 2 )\cdot (-1) + 1\cdot e^{a+2-x}
= e^{a+2-x}\cdot ( a + 2 - x + 1 )
= ( a + 3 - x )\cdot e^{a+2-x}

Wenn die dritte Ableitung am möglichen Wendepunkt ungleich Null ist, liegt ein Wendepunkt vor.

f_a^{'''} ( a + 2 ) = ( a + 3 - ( a + 2 ))\cdot e^{a + 2 - ( a + 2 )}
= ( a + 3 - a - 2 ) \cdot e^{a + 2 - a - 2 }
= 1\cdot e^{0}
= 1\;
> 0\;


\rightarrow WP ( a + 2 / 2 )\;

Von „http://rmg.zum.de/index.php?title=Facharbeit_Andre_Etzel/Teilaufgabe_a/Lösung:_Wendepunkte&oldid=28254“