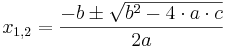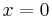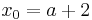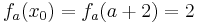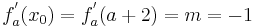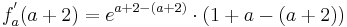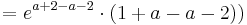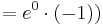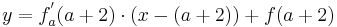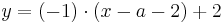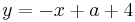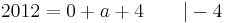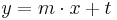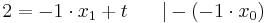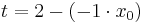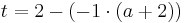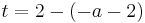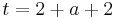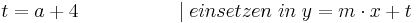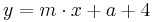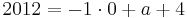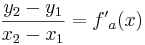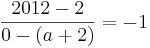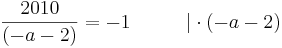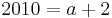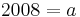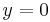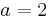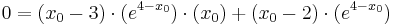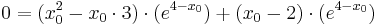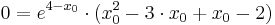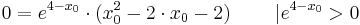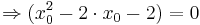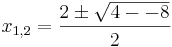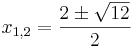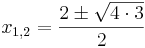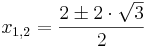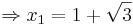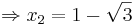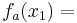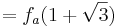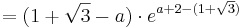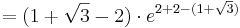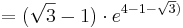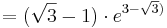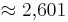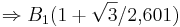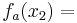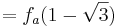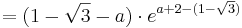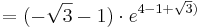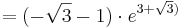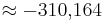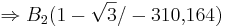Lösung von Teilaufgabe c
Aus RMG-Wiki
Inhaltsverzeichnis[Verbergen] |
Tangente im Punkt Wa( a + 2 / 2 ) an Gfa mit dem Schnittpunkt A (0 / 2012 )
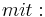
Lösung; Tangentengleichung
Tangentengleichung: siehe Formelsammlung Seite 58
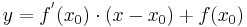
Lösung; Fußweg
Lösung; Clever
Berechnung derjenigen Punkte, für welche die Tangente an den Graphen von f2 durch den Ursprung verläuft
Verwendung der Tangentialgleichung
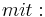
Lösen quadratischer Gleichungen mit Hilfe der Mitternachtsformel