Funktionen: Unterschied zwischen den Versionen
(→Teste dein Wissen) |
(→Teste dein Wissen) |
||
| Zeile 19: | Zeile 19: | ||
<br/> | <br/> | ||
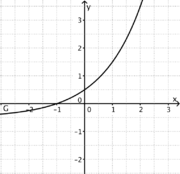
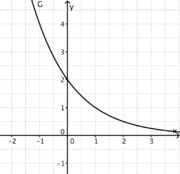
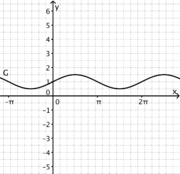
| − | + | '''1) Ordne Funktionstyp, Funktionsterm und Funktionsgraph passend zu. <br/>''' | |
| − | + | <div class="lueckentext-quiz"> | |
| − | '''1) Ordne Funktionstyp, Funktionsterm und Funktionsgraph passend zu. <br/> | + | {| class="wikitable center" |
| − | ''' | + | |- |
| − | + | | [[Datei:E1010.png|thumb]] || [[Datei:D1010.png|thumb]] || [[Datei:A.png|thumb]] || [[Datei:F1010.png|thumb]] || [[Datei:C1010.png|thumb]] || [[Datei:H1010.png|thumb]] || [[Datei:G1010.png|thumb]] || [[Datei:B1010.png|thumb]] | |
| − | + | ||
|- | |- | ||
| − | |<math> f_4(x)=0,5x^2+1 </math> || | + | |<strong> <math>f<sub>5</sub>(x)=0,5x+1</math> </strong> || <strong> <math> f_4(x)=0,5x^2+1 </math> </strong>|| <strong> <math> f_1(x)=x^3+1 </math> </strong> || <strong><math> f_6(x)=\frac {1}{x^2-4}-2 </math> </strong> || <strong> <math> f_3(x)=-0,2x^4+0,5x^2 </math> </strong>|| <strong> <math>f_8(x)=2^x-0,5</math> </strong> || <strong> <math>f_7(x)=2\cdot (\frac 1 2)^x</math> </strong>|| <strong> <math>f_2(x)=0,5sinx+1</math> </strong> |
|- | |- | ||
| − | | < | + | | <strong>Lineare Funktion</strong> || <strong>Quadratische Funktion</strong> || <strong>Ganzrationale Funktion</strong> || <strong> Gebrochen-rationale Funktion</strong> ||<strong> Ganzrationale Funktion</strong> ||<strong> Exponentialfunktion</strong> || <strong>Exponentialfunktion </strong> || <strong> Trigonometrische Funktion</strong> |
| − | | | + | |
| − | | < | + | |
| − | | | + | |
| − | | < | + | |
| − | + | ||
| − | | < | + | |
| − | + | ||
| − | | < | + | |
| − | + | ||
| − | | < | + | |
|} | |} | ||
Version vom 2. September 2014, 19:13 Uhr
|
Teste dein WissenUm die folgenden Aufgaben lösen zu können , solltest du mit diesen Funktionen umgehen können: 1) Ordne Funktionstyp, Funktionsterm und Funktionsgraph passend zu.
2) Entscheide, ob P(3/-6) auf dem Graphen der Funktion 3) Gib den Funktionsterm einer Geraden durch P(1/5) an, die parallel zur Geraden g: y=2x+4 verläuft.
4) Kreuze für
5) Gib das Verhalten der folgenden Funktionen für
|
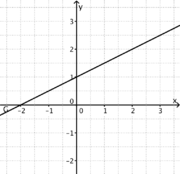
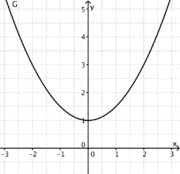

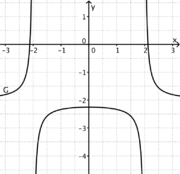
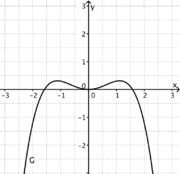
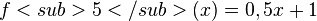
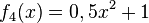


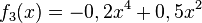
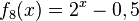

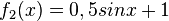
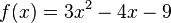 liegt.
(Nein, P liegt unterhalb von Gf)
(!Nein, P liegt oberhalb von Gf)
(!Ja, P liegt auf Gf)
liegt.
(Nein, P liegt unterhalb von Gf)
(!Nein, P liegt oberhalb von Gf)
(!Ja, P liegt auf Gf)
 die richtige Aussage an:
die richtige Aussage an:
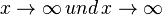 an.
an. 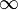 und "-u" für
und "-u" für 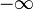 .
. 
