2006 V: Unterschied zwischen den Versionen
Aus RMG-Wiki
< LK Mathematik | Abitur
| Zeile 25: | Zeile 25: | ||
;Aufgabe 1 | ;Aufgabe 1 | ||
| − | In einem kartesischen Koordinatensystem des | + | In einem kartesischen Koordinatensystem des <math>\mathbb{R} </math><sup>3</sup> die |
| − | Ebene E: x<sub>2</sub> - x<sub>3</sub> - 1 = 0 , die Geradenschar g<sub>k</sub> : <math>\vec x = \begin{pmatrix} -k^2 \\ 0 \\ -1 \end{pmatrix} + \lambda \cdot\begin{pmatrix} 3 \\ 2 \\ 2 \end{pmatrix}</math> | + | Ebene E: x<sub>2</sub> - x<sub>3</sub> - 1 = 0 , die Geradenschar g<sub>k</sub> : <math>\vec x = \begin{pmatrix} -k^2 \\ 0 \\ -1 \end{pmatrix} + \lambda \cdot\begin{pmatrix} 3 \\ 2 \\ 2 \end{pmatrix}</math> und die Gerade h : <math>\vec x = \begin{pmatrix} 2 \\ 1 \\ 2 \end{pmatrix} + \mu \cdot\begin{pmatrix} 0 \\ -1 \\ 2 \end{pmatrix}</math> gegeben, wobei k, \lambda und \mu aus <math>\mathbb{R} </math> sind. |
Version vom 25. Februar 2010, 15:00 Uhr
|
|
In einem kartesischen Koordinatensystem des
|
Die Ebene E ist Tangentialebene an zwei Kukeln K1 und K2 mit dem Radius a)
|
Fehler beim Parsen(Syntaxfehler): \left( -k
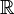 3 die
Ebene E: x2 - x3 - 1 = 0 , die Geradenschar gk :
3 die
Ebene E: x2 - x3 - 1 = 0 , die Geradenschar gk :  und die Gerade h :
und die Gerade h : 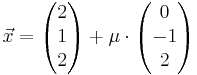 gegeben, wobei k, \lambda und \mu aus
gegeben, wobei k, \lambda und \mu aus 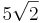 , deren Mittelpunkte M1 und M2 auf der Gerade h liegen.
, deren Mittelpunkte M1 und M2 auf der Gerade h liegen.

