Funktionsuntersuchungen: Unterschied zwischen den Versionen
| Zeile 19: | Zeile 19: | ||
<popup name="Lösung"> | <popup name="Lösung"> | ||
| − | [[Bild: | + | [[Bild:Graph5.1.png|450px|right]] |
D=<math>\mathbb{R}</math> <br /> | D=<math>\mathbb{R}</math> <br /> | ||
W=<math>\mathbb{R}</math> <br /> | W=<math>\mathbb{R}</math> <br /> | ||
| Zeile 40: | Zeile 40: | ||
<popup name="Lösung"> | <popup name="Lösung"> | ||
| − | [[Bild: | + | [[Bild:Graph5.2.png|450px|right]] |
D=<math>\mathbb{R}</math> | D=<math>\mathbb{R}</math> | ||
| Zeile 72: | Zeile 72: | ||
<popup name="Lösung"> | <popup name="Lösung"> | ||
| − | [[Bild: | + | [[Bild:Graph5.3.png|450px|right]] |
D=<math>\mathbb{R}</math> <br /> | D=<math>\mathbb{R}</math> <br /> | ||
W=<math>\mathbb{R}</math> <br /> | W=<math>\mathbb{R}</math> <br /> | ||
| Zeile 97: | Zeile 97: | ||
<popup name="Lösung"> | <popup name="Lösung"> | ||
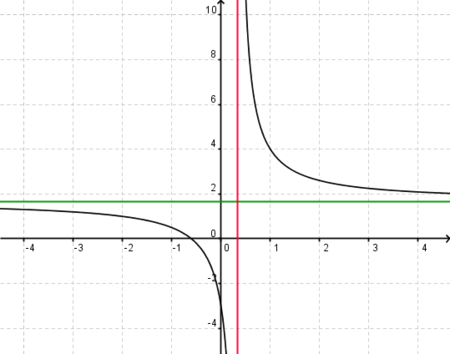
| − | [[Bild: | + | [[Bild:Graph5.4.png|450px|right]] |
Asymptoten: <br /> | Asymptoten: <br /> | ||
<math>\lim_{x\to \pm\infty}</math><math>{5x+3 \over 3x-1}</math>=<math>\lim_{x\to \pm\infty}</math><math>{x(5+3/x) \over x(3-1/x)}</math> =<math>{5 \over 3}</math> <br /> | <math>\lim_{x\to \pm\infty}</math><math>{5x+3 \over 3x-1}</math>=<math>\lim_{x\to \pm\infty}</math><math>{x(5+3/x) \over x(3-1/x)}</math> =<math>{5 \over 3}</math> <br /> | ||
| Zeile 116: | Zeile 116: | ||
<popup name="Lösung"> | <popup name="Lösung"> | ||
| − | [[Bild: | + | [[Bild:Graph5.5.png|450px|right]] |
<br /> <br /> | <br /> <br /> | ||
D=<math>\mathbb{R}</math> <br /> <br /> | D=<math>\mathbb{R}</math> <br /> <br /> | ||
| Zeile 132: | Zeile 132: | ||
<popup name="Lösung"> | <popup name="Lösung"> | ||
| − | [[Bild: | + | [[Bild:Graph5.6.png|450px|right]] |
<br /> <br /> | <br /> <br /> | ||
D=<math>\mathbb{R}</math> <br /> <br /> | D=<math>\mathbb{R}</math> <br /> <br /> | ||
Version vom 23. Januar 2010, 13:49 Uhr
FunktionsuntersuchungenLineare Funktionen
Quadratische FunktionenGegeben ist die Funktion g(x)=x2+3x-2. Bestimme zu dieser Funktion Definitions- und Wertemenge, die Nullstellen und den Scheitel. Beschreibe den Verlauf des Graphen und plotte die Funktion mit GeoGebra. Vergleiche anschließend die rechnerischen Ergebnisse mit der Zeichnung.
Ganzrationale FunktionenGegeben ist die Funktion h(x)=2x7-2x6+4x4-4x3. Bestimme zu dieser Funktion Definitionsbereich, Wertemenge und die Nullstellen. Untersuche die Funktion außerdem auf ihr Verhalten im Unendlichen. Kontrolliere deine Ergebnisse wieder mit GeoGebra.
Gebrochen rationale FunktionenBestimme zu der Funktion j(x)=
Trigonometrische FunktionenGegeben ist die Funktion k(x)=2cosx. Bestimme den Definitionsbereich, die Wertemenge, die Amplitude und die Nullstellen.
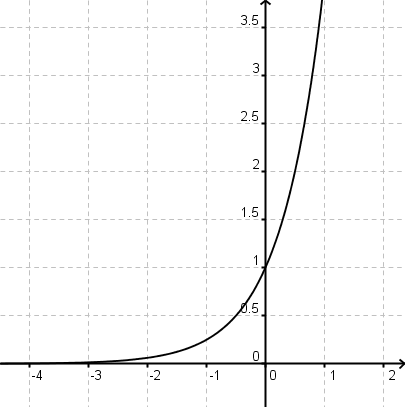
ExponentialfunktionenGegeben ist die Funktion l(x)=4x. Bestimme die Definitionsmenge, den Wertebereich, die Asymptote in x-Richtung und beschreibe den Verlauf des Graphen. Vergleiche deine Ergebnisse mit dem Graphen, den dir GeoGebra liefert.
WurzelfunktionenGegeben ist die Funktion f(x)= |
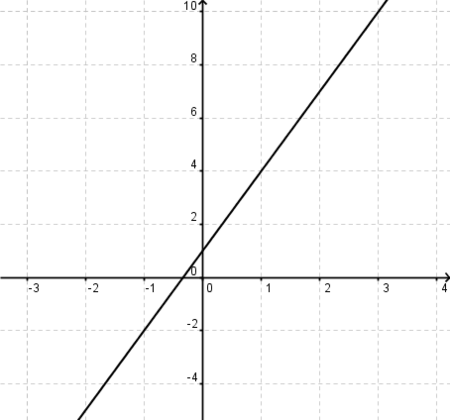
0+1=1 
P(0/1) 
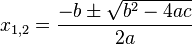
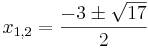
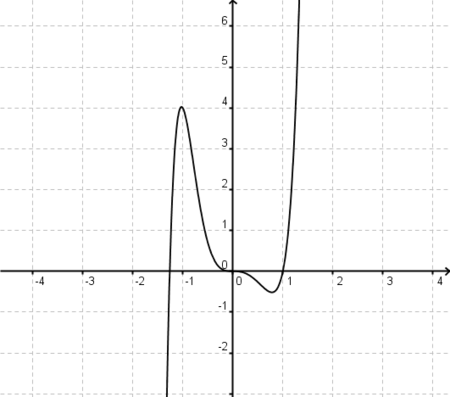
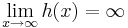
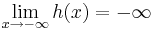
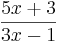die Asymptoten. Folgere daraus die Definitions- und Wertemenge. Berechne außerdem die Nullstelle der Funktion.
=
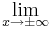
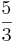
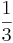
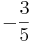
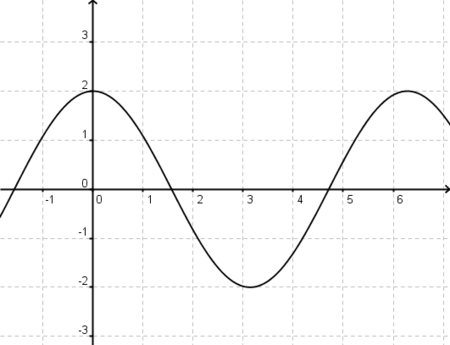
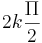
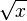. Bestimme die Definitionsmenge und die Nullstelle.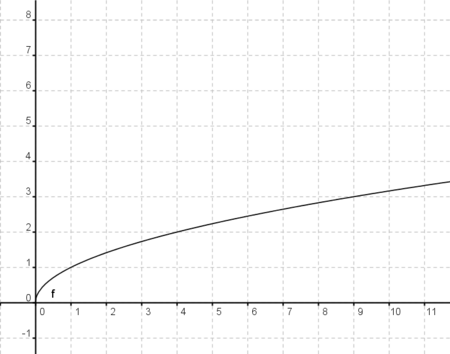 
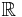+ da unter der Wurzel keine negativen Werte stehen dürfen.
