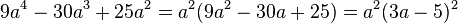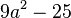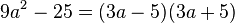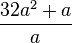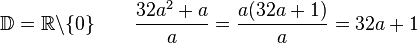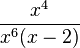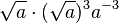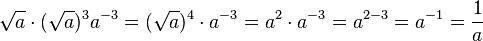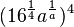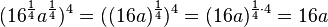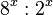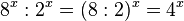Term und Zahl: Unterschied zwischen den Versionen

Aus RMG-Wiki
Wechseln zu: Navigation, Suche
K (typo)
 
(16 dazwischenliegende Versionen von einem Benutzer werden nicht angezeigt)
Zeile 1: Zeile 1:
 +
<br />
 +
__NOTOC__
 +
<div style="background: #ABAABF">
 +
 +
 +
 +
 +
<center><table border=0 width="800px" cellpadding=5 cellspacing=5>
 +
<tr><td  width="800px" valign="top">
 +
 +
 +
{|
 +
|width="70%"|
 +
 
== Teste dein Wissen==
 
== Teste dein Wissen==
 
   
 
   
−
 
 
<br />
 
<br />
−
<quiz>
+
'''1. Vereinfache folgende Terme soweit wie möglich.'''<br />
−
{1.Vereinfache folgende Terme soweit wie möglich.
+
<math>\frac48 a-(  \frac24 a- \frac93 b) =</math>  
−
| type="{}" }
+
<popup name="Lösung">
−
<math>\frac48 a-(  \frac24 a- \frac93 b) =</math> { 3b }
+
<math>=\frac48 a- \frac24 a+ \frac93 b=3b </math>  
−
<math> -x(-1-x)-(3+x)(4-x)+13=</math> { 1 }
+
</popup> <br/>
−
</quiz>
+
  
 +
<math> -x(-1+x)-(3+x)(4-x)+13=</math>
 +
<popup name="Lösung">
 +
<math> =x-x^2-(12-3x+4x-x^2)+13=x-x^2-12-x+x^2+13=1</math>
 +
</popup> <br />
  
−
2.Setze im ersten Term die Klammern so, dass eine richtige Termumformung entsteht. <br />
+
'''2. Setze im ersten Term die Klammern so, dass eine richtige Termumformung entsteht. <br />'''
 
<math>-2a^2+4a \cdot 2 - a = 8a</math>
 
<math>-2a^2+4a \cdot 2 - a = 8a</math>
 
<popup name="Lösung">
 
<popup name="Lösung">
Zeile 21: Zeile 37:
 
</popup> <br />
 
</popup> <br />
  
−
 
+
'''3. Klammere den größtmöglichen Faktor aus.<br/> '''
−
3.Klammere den größtmöglichen Faktor aus.<br/>  
+
 
<math> 12xy^2-18xy+15x^2y</math>
 
<math> 12xy^2-18xy+15x^2y</math>
 
<popup name = "Lösung">
 
<popup name = "Lösung">
Zeile 32: Zeile 47:
 
</math></popup><br/>
 
</math></popup><br/>
  
 +
|width="10%"|
  
−
4.Faktorisiere den Term (mit Hilfe der binomischen Formeln)<br/>
+
|valign="top"|
 +
 
 +
== Knicktests ==
 +
<br/>
 +
<br />
 +
<br />
 +
<br />
 +
[[Datei:1 AB1 Terme.pdf|thumb|Knicktest Termumformungen, Faktorisieren|200px|zentriert]]
 +
 
 +
|}
 +
 
 +
<br />
 +
<br />
 +
 
 +
{|
 +
|width="70%"|
 +
 
 +
'''4. Faktorisiere den Term (mit Hilfe der binomischen Formeln).<br/>'''
 
<math> 9a^2-30a+25</math>
 
<math> 9a^2-30a+25</math>
 
<popup name = "Lösung">
 
<popup name = "Lösung">
Zeile 39: Zeile 72:
 
<math>9a^4-30a^3+25a^2 </math>
 
<math>9a^4-30a^3+25a^2 </math>
 
<popup name = "Lösung">
 
<popup name = "Lösung">
 +
<math> 9a^4-30a^3+25a^2=(3a^2-5a)^2  </math> 2. binomische Formel <br/>
 
<math> 9a^4-30a^3+25a^2=a^2(9a^2-30a+25)=a^2(3a-5)^2 </math> <br/> a<sup>2</sup> ausklammern +2.binomische Formel
 
<math> 9a^4-30a^3+25a^2=a^2(9a^2-30a+25)=a^2(3a-5)^2 </math> <br/> a<sup>2</sup> ausklammern +2.binomische Formel
 
</popup><br/>
 
</popup><br/>
Zeile 45: Zeile 79:
 
<math> 9a^2-25=(3a-5)(3a+5)</math><br/> 3.binomische Formel
 
<math> 9a^2-25=(3a-5)(3a+5)</math><br/> 3.binomische Formel
 
</popup><br/>
 
</popup><br/>
 +
|width="10%"|
 +
|[[Datei:1 AB2 BinFormeln.pdf|thumb|Knicktest Binomische Formeln|200px|zentriert]]
 +
|}
 +
<br />
 +
<br />
  
−
 
+
{|
−
5. Gib die Definitionsmenge an und vereinfache soweit wie möglich<br/>
+
|width="70%"|
 +
'''5. Gib die Definitionsmenge an und vereinfache soweit wie möglich.<br/>'''
 
<math> \frac{32a^2+a}{a}</math>
 
<math> \frac{32a^2+a}{a}</math>
 
<popup name = "Lösung">
 
<popup name = "Lösung">
Zeile 57: Zeile 97:
 
<math>\mathbb{D}  =\mathbb{R}  \backslash \{0;2\} \qquad \frac{x^4}{x^6(x-2)}= \frac{1}{x^2(x-2)}  
 
<math>\mathbb{D}  =\mathbb{R}  \backslash \{0;2\} \qquad \frac{x^4}{x^6(x-2)}= \frac{1}{x^2(x-2)}  
 
</math></popup><br/>
 
</math></popup><br/>
 +
|width="10%"|
 +
|[[Datei:1 AB4 Bruchterme.pdf|thumb|Knicktest Bruchterme|200px|zentriert]]
 +
|}
 +
<br />
 +
<br />
  
−
 
+
{|
−
6) Vereinfache soweit wie möglich (a>0) <br/>
+
|width="70%"|
 +
'''6. Vereinfache soweit wie möglich (a>0) <br/>'''
 
<math> \sqrt a \cdot (\sqrt a)^3 a^{-3} </math>
 
<math> \sqrt a \cdot (\sqrt a)^3 a^{-3} </math>
 
<popup name = "Lösung">
 
<popup name = "Lösung">
Zeile 72: Zeile 118:
 
<math>  8^x:2^x=(8:2)^x=4^x
 
<math>  8^x:2^x=(8:2)^x=4^x
 
</math></popup><br/>
 
</math></popup><br/>
 +
|width="10%"|
 +
|[[Datei:1_AB3_Potenzen.pdf|thumb|Knicktest Potenzen und Wurzeln|200px|zentriert]]
 +
|}
 +
 +
[[Mathematik_Grundwissen_10|Zurück zur Übersicht]]
 +
</td></tr></table></center>
 +
 +
 +
 +
</div>

Aktuelle Version vom 25. September 2014, 17:57 Uhr





Teste dein Wissen


1. Vereinfache folgende Terme soweit wie möglich.
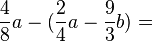
\frac48 a-( \frac24 a- \frac93 b) =


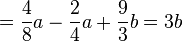
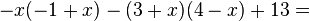
-x(-1+x)-(3+x)(4-x)+13=


2. Setze im ersten Term die Klammern so, dass eine richtige Termumformung entsteht.
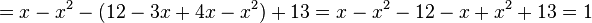
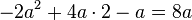
-2a^2+4a \cdot 2 - a = 8a


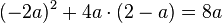
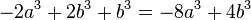
-2a^3+2b^3+b^3 = -8a^3+4b^3


3. Klammere den größtmöglichen Faktor aus.
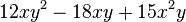
 12xy^2-18xy+15x^2y


4(x+y)+5(2x+2y)


Knicktests





Knicktest Termumformungen, Faktorisieren



4. Faktorisiere den Term (mit Hilfe der binomischen Formeln).
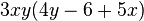
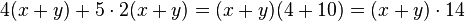
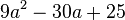
 9a^2-30a+25


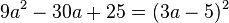
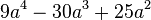
9a^4-30a^3+25a^2


9a^2-25


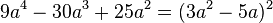Knicktest Binomische Formeln



5. Gib die Definitionsmenge an und vereinfache soweit wie möglich.
 \frac{32a^2+a}{a}


\frac{x^4}{x^6(x-2)}


Knicktest Bruchterme



6. Vereinfache soweit wie möglich (a>0)
\sqrt a \cdot (\sqrt a)^3 a^{-3}


(16^{\frac1 4} a^{\frac1 a})^4


8^x:2^x


Knicktest Potenzen und Wurzeln

Zurück zur Übersicht


Von „http://rmg.zum.de/index.php?title=Mathematik_Grundwissen_10/Term_und_Zahl&oldid=58701“