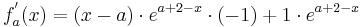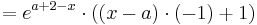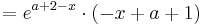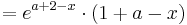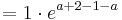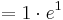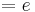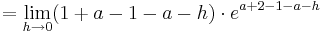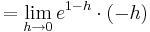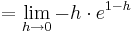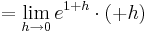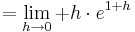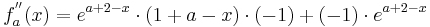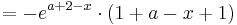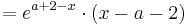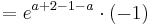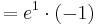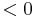Lösung: lokale Extrempunkte: Unterschied zwischen den Versionen

Aus RMG-Wiki
Wechseln zu: Navigation, Suche
(→‎1. Möglichkeit)
(→‎1. Möglichkeit; H-Methode)
 
(28 dazwischenliegende Versionen von einem Benutzer werden nicht angezeigt)
Zeile 12: Zeile 12:
 
[[http://de.wikipedia.org/wiki/Produktregel Hilfe zur Produktregel]]
 
[[http://de.wikipedia.org/wiki/Produktregel Hilfe zur Produktregel]]
  
−
                          <math> f_a^{'}(x) = ( x - a )\cdot e^{a+2-x}\cdot( -1 ) + 1\cdot e^{a+2-x}</math><br />
+
:                          <math> f_a^{'}(x) = ( x - a )\cdot e^{a+2-x}\cdot( -1 ) + 1\cdot e^{a+2-x}</math><br />
−
                                <math>= e^{a+2-x}\cdot (( x - a )\cdot (-1) + 1 )</math><br />
+
:::                                <math>= e^{a+2-x}\cdot (( x - a )\cdot (-1) + 1 )</math><br />
−
                                <math> = e^{a+2-x}\cdot ( -x + a + 1 )</math><br />
+
:::                                <math> = e^{a+2-x}\cdot ( -x + a + 1 )</math><br />
−
                                <math>= e^{a+2-x}\cdot ( 1 + a - x )</math><br />
+
:::                                <math>= e^{a+2-x}\cdot ( 1 + a - x )</math><br />
  
  
−
Der/Die Extrempunkt/e können an der Stelle liegen, an der die erste Ableitung der Funktion gliech Null ist. Die erste Ableitung einer Funktion zeigt das Steigungsverhalten dieser an. Wenn dieses gleich Null ist, besitzt die Funktion eine waagrechte Tangent an dieser Stelle.
+
Der/Die Extrempunkt/e können an der Stelle liegen, an der die erste Ableitung der Funktion gleich Null ist. Die erste Ableitung einer Funktion zeigt das Steigungsverhalten dieser an. Wenn dieses gleich Null ist, besitzt die Funktion eine waagrechte Tangente an dieser Stelle.
−
Dass heißt es könnte ein Extrempunkt(Maximum<u>^</u>Hochpunkt und/oder Minimum <u>^</u> Tiefpunkt)auftreten.Dies muss jedoch erst mit der zweiten Ableitung oder mit dem Monotonieverhalten der Funktion überprüft werden, da auch ein Terassenpunktauftreten könnte.
+
Das heißt, es könnte ein Extrempunkt(Maximum / Hochpunkt und/oder Minimum / Tiefpunkt)auftreten.Dies muss jedoch erst mit der zweiten Ableitung oder mit dem Monotonieverhalten der Funktion überprüft werden, da auch ein Terassenpunkt auftreten könnte.
  
  
−
                          <math> f_a^{'}(x) = 0  \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;  |e^{a+2-x} > 0 </math><br />
+
::::<math> f_a^{'}(x) = 0  \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;  |e^{a+2-x} > 0 </math><br />
−
                    <math>( 1 + a - x ) = 0  \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;  |- 1 ; - a</math> <br />
+
::<math>( 1 + a - x ) = 0  \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;  |- 1 ; - a</math> <br />
−
                              <math>-x = -1 + a \;\;\;\;\;\;\;\;\;\;\;\;\;\;\ |\cdot (-1)</math><br />
+
::::<math>-x = -1 + a \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;|\cdot (-1)</math><br />
−
                                <math>x = 1 + a \;</math><br />
+
::::<math>x = 1 + a \;</math><br />
  
 +
 +
X-Koordinate des möglichen Extrempunkts in die Funktion einsetzen, um die y-Koordinate zu erhalten.
 
   
 
   
 +
 +
:              <math> y = f_a ( 1 + a ) = ( 1 + a - a )\cdot e^{a + 2 - ( 1 + a )}</math> <br />
 +
::                              <math> =  1\cdot e^{a + 2 - 1 - a }</math>  <br />
 +
::                            <math> = 1\cdot e^{1}  </math><br />
 +
 +
::                              <math> = e \;</math><br />
 +
 
   
 
   
−
              <math> y = f_a ( 1 + a ) = ( 1 + a - a )\cdot e^{a + 2 - ( 1 + a )}</math> <br />
+
:::<math>\rightarrow</math> Möglicher Extrempunkt:  <math>( 1 + a / e )\;</math>
−
                              <math> =  1\cdot e^{a + 2 - 1 - a }</math>  <br />
+
−
                              <math> = 1\cdot e^{1}  </math><br />
+
−
                              <math> = e \;</math><br />
+
−
+
−
<math>\rightarrow</math> Möglicher Extrempunkt:  <math>( 1 + a / e )\;</math>
+
  
  
Zeile 41: Zeile 45:
  
  
−
==== 1. Möglichkeit ====
+
==== 1. Möglichkeit; H-Methode ====
  
−
H-Methode<br />
 
−
Vorzeichenwechsel (VZW) des Monotonieverhaltens der Funktion
 
  
−
<math>f_a^{'}( 1 + a + h ) = ( 1 + a -( 1 + a + h )\cdot e^{a + 2 - ( 1 + a + h)}</math><br />
+
Wenn es einen Vorzeichenwechsel (VZW) des Monotonieverhaltens der Funktion am möglichen Extrempunkt gibt, kann man von einem Extrempunkt sprechen.
−
                                        <math>= ( 1 + a - 1 - a - h )\cdot e^{a + 2 - 1 - a - h}</math> <br />
+
−
                                        <math>= e^{1 - h}\cdot ( -h )</math><br />
+
−
                                        <math>= -h\cdot e^{1 - h}</math><br />
+
−
+
−
<math>\lim_{h\to 0} f_a^{'} ( 1 + a + h ) < 0 </math><br />
+
  
−
<math>\rightarrow</math> An der Stelle  <math>f_a^{'}( 1 + a + h )\;</math> fällt der Graph (I)
+
'''I.'''<br />
 +
<math>\lim_{h\to 0}f_a^{'}( 1 + a + h ) = \lim_{h\to 0}( 1 + a -( 1 + a + h )\cdot e^{a + 2 - ( 1 + a + h)}</math><br />
 +
::::::                                        <math>=\lim_{h\to 0} ( 1 + a - 1 - a - h )\cdot e^{a + 2 - 1 - a - h}</math> <br />
 +
::::::                                        <math>= \lim_{h\to 0}e^{1 - h}\cdot ( -h )</math><br />
 +
::::::                                        <math>= \lim_{h\to 0}-h\cdot e^{1 - h}</math><br />
 +
<br />
 +
<math>\lim_{h\to 0} f_a^{'} ( 1 + a + h ) < 0 </math><br />
  
 +
<math>\rightarrow</math>  An der Stelle  <math>\lim_{h\to 0}f_a^{'}( 1 + a + h )\;</math> fällt der Graph (I)
  
  
−
<math>f_a^{'} ( 1 + a - h ) = ( 1 + a -( 1 + a - h ) e^{a + 2 - ( 1 + a - h)}</math><br />
 
−
                                        <math>= ( 1 + a - 1 - a + h )\cdot e^{a + 2 - 1 - a + h)}</math><br />
 
−
                                        <math> =  e^{1+ h}\cdot ( +h )</math><br />
 
−
                                        <math>= +h\cdot e^{1+ h}</math><br />
 
−
 
−
<math>\lim_{h\to 0} f_a^{'}  ( 1 + a - h ) > 0 </math><br />
 
  
−
<math>\rightarrow</math>  An der Stelle  <math>f_a^{'}( 1 + a - h )\;</math> steigt der Graph (II)
+
'''II.'''<br />
 +
<math>\lim_{h\to 0}f_a^{'} ( 1 + a - h ) = \lim_{h\to 0}( 1 + a -( 1 + a - h ) e^{a + 2 - ( 1 + a - h)}</math><br />
 +
::::::                                        <math>= \lim_{h\to 0}( 1 + a - 1 - a + h )\cdot e^{a + 2 - 1 - a + h)}</math><br />
 +
::::::                                        <math> = \lim_{h\to 0} e^{1+ h}\cdot ( +h )</math><br />
 +
::::::                                        <math>= \lim_{h\to 0}+h\cdot e^{1+ h}</math><br />
 +
<br />
 +
<math>\lim_{h\to 0} f_a^{'}  ( 1 + a - h ) > 0 </math><br />
 +
 
 +
<math>\rightarrow</math>  An der Stelle  <math>\lim_{h\to 0}f_a^{'}( 1 + a - h )\;</math> steigt der Graph (II)
  
  
 
Aus (I) und (II) folgt:<br />
 
Aus (I) und (II) folgt:<br />
 
VZW bei <math>x = 1 + a\;</math><br />
 
VZW bei <math>x = 1 + a\;</math><br />
−
<math>\Rightarrow</math>  Extrempunkt bei <math>( 1 + a / e )\;</math>  
+
<math>\Rightarrow</math>  Extrempunkt bei <math>( 1 + a / e )\;</math><br />
 +
Bei diesem Extrempunkt handelt es sich um ein Maximum, da das Monotonieverhalten des Graphen von <math>f_a\,</math> kurz vor dem Extrempunkt streng monoton steigend und kurz nach dem Extrempunkt streng monoton fallend ist.
  
−
 
+
<u>zur Verdeutlichung:</u>
−
<u>zur Verdeutlichung</u>
+
  
 
{| class="wikitable centersortable"
 
{| class="wikitable centersortable"
Zeile 119: Zeile 125:
 
|  
 
|  
 
|}
 
|}
 +
 +
::::<math>\;\;\;\nearrow \;\;\;\;\;\;\;\;\; \searrow</math><br />
 +
  
  
 
<math>\rightarrow Maximum \;( 1 + a / e )\;</math>
 
<math>\rightarrow Maximum \;( 1 + a / e )\;</math>
  
−
==== 2. Möglichkeit ====
+
==== 2. Möglichkeit; 2.Ableitung ====
  
 
Überprüfung durch die zweite Ableitung  [[http://de.wikipedia.org/wiki/Produktregel Hilfe zur Produktregel]]
 
Überprüfung durch die zweite Ableitung  [[http://de.wikipedia.org/wiki/Produktregel Hilfe zur Produktregel]]
  
  
−
<math>y = f_a (x) = ( x - a )\cdot e^{a + 2 - x}</math>
+
:<math>y = f_a (x) = ( x - a )\cdot e^{a + 2 - x}</math><br />
  
−
    <math>f_a^{'}(x) = e^{a + 2 - x}\cdot ( 1 + a - x )</math><br />
 
  
−
   <math>f_a^{''}(x) = e^{a + 2 - x}\cdot ( 1 + a - x )\cdot ( -1 ) +  ( -1 )\cdot e^{a + 2 - x}</math><br />
+
:   <math>f_a^{'}(x) = e^{a + 2 - x}\cdot ( 1 + a - x )</math><br />
−
                                  <math>= -e^{a + 2 - x}\cdot ( 1 + a - x + 1 )</math><br />
+
−
                                  <math>= e^{a + 2 - x}\cdot ( x - a - 2 )</math>
+
  
−
Wenn die zweite Ableitung an dem möglichen Extrempunkt größer als Null ist hat man ein Minimum, wenn sie kleiner Null ist ein Maximum, bei gleich Null könnte ein Terrassenpunkt auftreten.
 
  
−
<math>f_a^{''} ( 1 + a ) = e^{a + 2 - ( 1 + a )}\cdot ( ( 1 + a ) - a - 2 )</math>
+
:  <math>f_a^{''}(x) = e^{a + 2 - x}\cdot ( 1 + a - x )\cdot ( -1 ) +  ( -1 )\cdot e^{a + 2 - x}</math><br />
−
                                      <math>= e^{a + 2 - 1 - a }\cdot ( -1 )</math>
+
:::                                  <math>= -e^{a + 2 - x}\cdot ( 1 + a - x + 1 )</math><br />
−
                                      <math>= e^{1}\cdot ( -1 )</math>  
+
:::                                  <math>= e^{a + 2 - x}\cdot ( x - a - 2 )</math><br />
−
                                      <math> < 0\;</math>
+
  
 +
 +
Falls die zweite Ableitung an dem möglichen Extrempunkt größer als Null ist, tritt ein Minimum auf. Falls sie kleiner als Null ist, handelt es sich um ein Maximum, bei gleich Null könnte ein Terrassenpunkt auftreten.<br />
 +
 +
 +
:<math>f_a^{''} ( 1 + a ) = e^{a + 2 - ( 1 + a )}\cdot ( ( 1 + a ) - a - 2 )</math>
 +
::::                                      <math>= e^{a + 2 - 1 - a }\cdot ( -1 )</math>
 +
::::                                      <math>= e^{1}\cdot ( -1 )</math>
 +
::::                                      <math> < 0\;</math><br />
 +
<br />
 
<math>\rightarrow  Max ( 1 + a / e )</math>
 
<math>\rightarrow  Max ( 1 + a / e )</math>
 +
 +
 +
 +
--[[Benutzer:Andre Etzel|Andre Etzel]] 23:49, 22. Jan. 2010 (UTC)

Aktuelle Version vom 26. Januar 2010, 19:38 Uhr

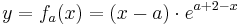y = f_a (x) = ( x - a )\cdot e^{a+2-x} mit x\in R ; a\in R


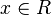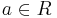
Inhaltsverzeichnis

lokale Extrempunkte

Damit man Extrempunkte einer Funktion finden kann, braucht man ihre erste Ableitung

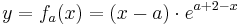
y = f_a (x) = ( x - a )\cdot e^{a+2-x}

Um die erste Ableitung zu bekommen, muss man hier die Produktregel verwenden [Hilfe zur Produktregel]

f_a^{'}(x) = ( x - a )\cdot e^{a+2-x}\cdot( -1 ) + 1\cdot e^{a+2-x}
= e^{a+2-x}\cdot (( x - a )\cdot (-1) + 1 )
= e^{a+2-x}\cdot ( -x + a + 1 )
= e^{a+2-x}\cdot ( 1 + a - x )


Der/Die Extrempunkt/e können an der Stelle liegen, an der die erste Ableitung der Funktion gleich Null ist. Die erste Ableitung einer Funktion zeigt das Steigungsverhalten dieser an. Wenn dieses gleich Null ist, besitzt die Funktion eine waagrechte Tangente an dieser Stelle. Das heißt, es könnte ein Extrempunkt(Maximum / Hochpunkt und/oder Minimum / Tiefpunkt)auftreten.Dies muss jedoch erst mit der zweiten Ableitung oder mit dem Monotonieverhalten der Funktion überprüft werden, da auch ein Terassenpunkt auftreten könnte.


f_a^{'}(x) = 0 \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\; |e^{a+2-x} > 0
( 1 + a - x ) = 0 \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\; |- 1 ; - a
-x = -1 + a \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;|\cdot (-1)
x = 1 + a \;


X-Koordinate des möglichen Extrempunkts in die Funktion einsetzen, um die y-Koordinate zu erhalten.


y = f_a ( 1 + a ) = ( 1 + a - a )\cdot e^{a + 2 - ( 1 + a )}
= 1\cdot e^{a + 2 - 1 - a }
= 1\cdot e^{1}
= e \;


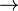\rightarrow Möglicher Extrempunkt: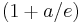 ( 1 + a / e )\;


Überprüfung des Extrempunkts

1. Möglichkeit; H-Methode

Wenn es einen Vorzeichenwechsel (VZW) des Monotonieverhaltens der Funktion am möglichen Extrempunkt gibt, kann man von einem Extrempunkt sprechen.

I.
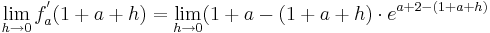
\lim_{h\to 0}f_a^{'}( 1 + a + h ) = \lim_{h\to 0}( 1 + a -( 1 + a + h )\cdot e^{a + 2 - ( 1 + a + h)}

=\lim_{h\to 0} ( 1 + a - 1 - a - h )\cdot e^{a + 2 - 1 - a - h}
= \lim_{h\to 0}e^{1 - h}\cdot ( -h )
= \lim_{h\to 0}-h\cdot e^{1 - h}


\lim_{h\to 0} f_a^{'} ( 1 + a + h ) < 0

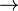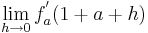\rightarrow An der Stelle \lim_{h\to 0}f_a^{'}( 1 + a + h )\; fällt der Graph (I)


II.
\lim_{h\to 0}f_a^{'} ( 1 + a - h ) = \lim_{h\to 0}( 1 + a -( 1 + a - h ) e^{a + 2 - ( 1 + a - h)}

= \lim_{h\to 0}( 1 + a - 1 - a + h )\cdot e^{a + 2 - 1 - a + h)}
= \lim_{h\to 0} e^{1+ h}\cdot ( +h )
= \lim_{h\to 0}+h\cdot e^{1+ h}


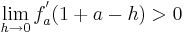
\lim_{h\to 0} f_a^{'} ( 1 + a - h ) > 0

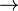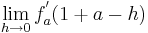\rightarrow An der Stelle \lim_{h\to 0}f_a^{'}( 1 + a - h )\; steigt der Graph (II)


Aus (I) und (II) folgt:
VZW bei 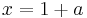x = 1 + a\;
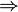\Rightarrow Extrempunkt bei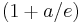 ( 1 + a / e )\;
Bei diesem Extrempunkt handelt es sich um ein Maximum, da das Monotonieverhalten des Graphen von 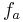f_a\, kurz vor dem Extrempunkt streng monoton steigend und kurz nach dem Extrempunkt streng monoton fallend ist.

zur Verdeutlichung:

Monotonieverhalten
x<1+a x=1+a x>1+a
ea + 2 - x + +
( 1 + a - x ) + -
fa' ( x ) + -
\;\;\;\nearrow \;\;\;\;\;\;\;\;\; \searrow


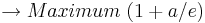
\rightarrow Maximum \;( 1 + a / e )\;

2. Möglichkeit; 2.Ableitung

Überprüfung durch die zweite Ableitung [Hilfe zur Produktregel]


y = f_a (x) = ( x - a )\cdot e^{a + 2 - x}


f_a^{'}(x) = e^{a + 2 - x}\cdot ( 1 + a - x )


f_a^{''}(x) = e^{a + 2 - x}\cdot ( 1 + a - x )\cdot ( -1 ) + ( -1 )\cdot e^{a + 2 - x}
= -e^{a + 2 - x}\cdot ( 1 + a - x + 1 )
= e^{a + 2 - x}\cdot ( x - a - 2 )


Falls die zweite Ableitung an dem möglichen Extrempunkt größer als Null ist, tritt ein Minimum auf. Falls sie kleiner als Null ist, handelt es sich um ein Maximum, bei gleich Null könnte ein Terrassenpunkt auftreten.


f_a^{''} ( 1 + a ) = e^{a + 2 - ( 1 + a )}\cdot ( ( 1 + a ) - a - 2 )
= e^{a + 2 - 1 - a }\cdot ( -1 )
= e^{1}\cdot ( -1 )
< 0\;


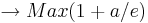
\rightarrow Max ( 1 + a / e )


--Andre Etzel 23:49, 22. Jan. 2010 (UTC)

Von „http://rmg.zum.de/index.php?title=Facharbeit_Andre_Etzel/Teilaufgabe_a/Lösung:_lokale_Extrempunkte&oldid=28251“