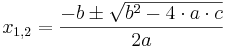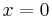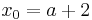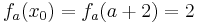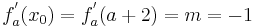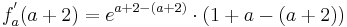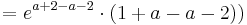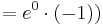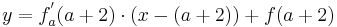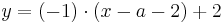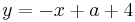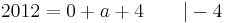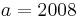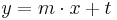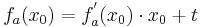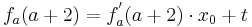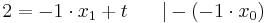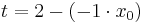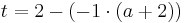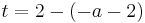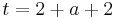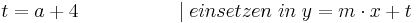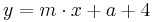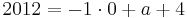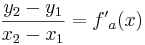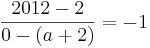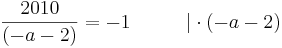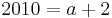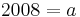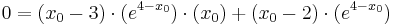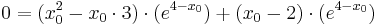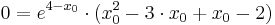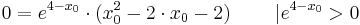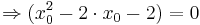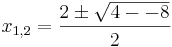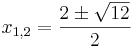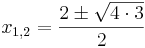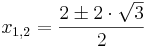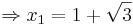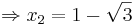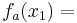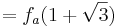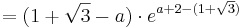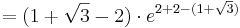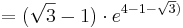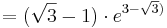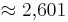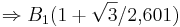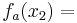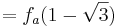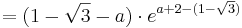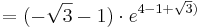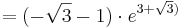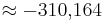Lösung von Teilaufgabe c: Unterschied zwischen den Versionen

Aus RMG-Wiki
Wechseln zu: Navigation, Suche
(→‎2. Lösung; Clever)
(→‎Berechnung derjenigen Punkte, für welche die Tangente an den Graphen von f2 durch den Ursprung verläuft)
 
(25 dazwischenliegende Versionen von einem Benutzer werden nicht angezeigt)
Zeile 1: Zeile 1:
−
== Tangente im Punkt W<sub>a</sub> ( a + 2 / 2 ) an G<sub>f<sub>a</sub></sub> mit dem Schnittpunkt A (0 / 2012 ) ==
+
== Tangente im Punkt W<sub>a</sub>( a + 2 / 2 ) an G<sub>f<sub>a</sub></sub> mit dem Schnittpunkt A (0 / 2012 ) ==
  
 +
<math>mit:\;</math><br />
 +
 +
:<math>x = 0\;</math><br />
 +
:<math>y = 2012\;</math><br />
 +
:<math>x_0 = a + 2\;</math><br />
 +
:<math>f_a( x_0 ) = f_a( a + 2 ) = 2\;</math><br />
 +
:<math>f^{'}_a( x_0 ) = f^{'}_a( a + 2 ) = m = -1\;</math><br />
  
−
=== 1. Lösung; Tangentengleichung ===
+
 +
:          <math>  f^{'}_a( a + 2 ) = e^{a + 2 - ( a + 2 )}\cdot ( 1 + a - ( a + 2 ) )</math><br />
 +
::::                      <math> = e^{a + 2 - a - 2 }\cdot ( 1 + a -  a - 2 ) )</math><br />
 +
::::                      <math> = e^{0}\cdot ( -1 ) )</math><br />
 +
::::<math>= -1\;</math><br />
  
−
Allgemeine Tangentengleichung: siehe Formelsammlung Seite ......<br />
 
  
−
'''y = f<sup>'</sup>( x<sub>0</sub> ) ( x - x<sub>0</sub> ) + f ( x<sub>0</sub> )'''<br />
+
=== Lösung; Tangentengleichung ===
  
 +
Tangentengleichung: siehe Formelsammlung Seite 58<br />
  
 +
<math>y = f^{'}( x_0 )\cdot ( x - x_0 ) + f ( x_0)</math><br />
  
−
mit:<br />
 
−
x = 0<br />
 
−
y = 2012<br />
 
−
x<sub>0</sub> = a + 2<br />
 
−
f<sub>a</sub>( x<sub>0</sub> ) = f<sub>a</sub>( a + 2 ) = 2<br />
 
−
f<sup>'</sup><sub>a</sub>( x<sub>0</sub> ) = f<sup>'</sup><sub>a</sub>( a + 2 ) = m = -1<br />
 
  
−
          f<sup>'</sup>( a + 2 ) = e<sup>a + 2 - ( a + 2 ) </sup> ( 1 + a - ( a + 2 ) )
+
::    <math>y = f^{'}_a( a + 2 )\cdot ( x - ( a + 2 )) + f ( a + 2 )</math><br />
−
                      = e<sup>a + 2 - a - 2 ) </sup> ( 1 + a - a - 2 ) )
+
::    <math>y = (-1)\cdot ( x - a - 2 ) + 2</math><br />
−
                      = e <sup>0</sup> ( -1 )
+
::    <math>y = -x + a + 2 + 2\;</math> <br />
−
                      = -1
+
::    <math>y = -x + a + 4\;</math> <br />
 +
:  <math>2012 = 0 + a + 4\;\;\;\;\;\;\;          | -4</math><br />
 +
::    <math>a = 2008\;</math><br />
  
−
    y = f<sup>'</sup>( a + 2 ) ( x - ( a + 2 )) + f ( a + 2 )
+
=== Lösung; Fußweg ===
−
    y = (-1) ( x - a - 2 ) + 2
+
−
    y = -x + a + 2 + 2
+
−
    y = -x + a + 4
+
−
  2012 = 0 + a + 4          / -4
+
−
    a = 2008
+
  
−
=== 2. Lösung; Fußweg ===
+
:::                  <math>  y = m\cdot x + t  </math><br />
 +
 +
::            <math>f_a( x_0 ) = f^{'}_a( x_0 )\cdot x_0 + t  </math><br /> 
 +
:        <math> f_a( a + 2 ) = f^{'}_a( a + 2 )\cdot x_0 + t</math><br />
 +
::::                <math>2 = -1\cdot x_1 + t \;\;\;\;\;\;          | - ( -1\cdot x_0)</math><br />
 +
::::                <math>t = 2 - ( -1\cdot x_0 )</math><br />
 +
::::                <math>t = 2 - ( -1\cdot ( a + 2 ))</math><br />
 +
::::                <math>t = 2 - ( -a - 2)\;</math><br />
 +
::::                <math>t = 2 + a + 2 \;</math><br />
 +
::::                <math>t = a + 4 \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;        |\;einsetzen\; in\; y = m\cdot x + t</math><br />
  
−
              y = m x + t 
 
−
        f<sub>a</sub>( x<sub>0</sub> ) = f<sup>'</sup><sub>a</sub>( x<sub>0</sub> ) x<sub>0</sub> + t   
 
−
    f<sub>a</sub>( a + 2 ) = f<sup>'</sup><sub>a</sub>( a + 2 ) x<sub>0</sub> + t
 
−
              2 = -1 x<sub>0</sub> + t      / - ( -1 x<sub>0</sub> )
 
−
              t = 2 - ( -1 x<sub>0</sub> )
 
−
              t = 2 - ( -1 ( a + 2 ))
 
−
              t = 2 - ( -a - 2)
 
−
              t = 2 + a + 2
 
−
              t = a + 4          einsetzen in y = m x + t
 
  
−
  y = m x + a + 4
+
::    <math>y = m\cdot x + a + 4</math><br />
−
  2012 = -1*0 + a + 4
+
: <math> 2012 = -1\cdot 0 + a + 4</math><br />
−
  2012 = a + 4  
+
:  <math>2012 = a + 4 \;</math><br />
−
    a = 2008
+
::    <math>a = 2008\;</math><br />
  
−
=== 3. Lösung; Clever ===
+
=== Lösung; Clever ===
  
−
<math>\frac{y - y}{x - x}</math> = '''f <sup> '</sup><sub>a</sub>'''
+
:: <math>\frac{y_2 - y_1}{x_2 - x_1} = f{'}_a ( x )</math><br />
 +
 +
: <math>\frac{2012 - 2}{0 - ( a + 2 )} = -1 </math><br />
 +
 +
:: <math>\frac{2010}{(-a - 2 )} = -1 \;\;\;\;\;\;\;\;\;\;| \cdot( -a - 2 )</math><br />
 +
 +
:::: <math>2010 = a + 2\;</math><br />
 +
:::: <math>2008 = a\;</math><br />
  
−
<math>\frac{2012 - 2}{0 - ( a + 2 )}</math> = -1
+
== Berechnung derjenigen Punkte, für welche die Tangente an den Graphen von f<sub>2</sub> durch den Ursprung verläuft ==
  
−
<math>\frac{2010}{-a - 2 )}</math> = -1          | *( a + 2 )
 
  
−
2010 = a + 2
+
=== Verwendung der Tangentialgleichung ===
−
  2008 = a
+
 
 +
: <math>y = f^{'}( x_0)\cdot ( x - x_0 ) + f ( x_0 )</math><br />
 +
 
 +
:<math> y = ( x_0 - a - 1 )\cdot ( -e^{a + 2 - x_0})\cdot ( x - x_0 ) + ( x_0 - a )\cdot e^{a + 2 - x_0})</math><br />
 +
 
 +
 
 +
<math>mit:\;</math><br />
 +
: <math>y = 0\;</math><br />
 +
: <math>x = 0\;</math><br />
 +
: <math>a = 2\;</math><br />
 +
 
 +
 
 +
 
 +
::    <math>0 = ( x_0 - 3 )\cdot ( -e^{4 - x_0} )\cdot ( -x_0 ) + ( x_0 - 2 )\cdot ( e^{4 - x_0} )</math>
 +
   
 +
::    <math> 0 = ( x_0 - 3 )\cdot ( e^{4 - x_0} )\cdot ( x_0 ) + ( x_0 - 2 )\cdot ( e^{4 - x_0} )</math>
 +
 +
::    <math> 0 = ( x_0^{2} - x_0\cdot 3 )\cdot ( e^{4 - x_0} ) + ( x_0 - 2 )\cdot ( e^{4 - x_0} )</math>
 +
 +
::    <math> 0 = e^{4 - x_0}\cdot ( x_0^{2} - 3\cdot x_0 +  x_0 - 2 )</math>
 +
 +
::    <math> 0 = e^{4 - x_0}\cdot ( x_0^{2} - 2\cdot x_0 - 2 )\;\;\;\;\;\;\;\;|e^{4 - x_0}>0</math>
 +
 +
:: <math>\Rightarrow ( x_0^{2} - 2\cdot x_0 - 2 ) = 0</math>
 +
 +
Lösen quadratischer Gleichungen mit Hilfe der [http://de.wikipedia.org/wiki/Mitternachtsformel?title=Mitternachtsformel&redirect=no Mitternachtsformel] <math> x_{1,2} = \frac{-b\pm\sqrt{b^{2}-4\cdot a\cdot c}}{2a}</math>
 +
 +
: <math> x_{1,2} = \frac{2\pm\sqrt{4--8}}{2}</math><br />
 +
: <math> x_{1,2} = \frac{2\pm\sqrt{4+8}}{2}</math><br />
 +
: <math> x_{1,2} = \frac{2\pm\sqrt{12}}{2}</math><br />
 +
: <math> x_{1,2} = \frac{2\pm\sqrt{4\cdot 3}}{2}</math><br />
 +
: <math> x_{1,2} = \frac{2\pm2\cdot\sqrt{3}}{2}</math><br />
 +
: <math> x_{1,2} = {1\pm\sqrt{3}}</math><br />
 +
 
 +
 
 +
: <math>\Rightarrow x_{1} = {1 + \sqrt{3}}</math><br />
 +
 
 +
: <math>\Rightarrow x_{2} = {1 - \sqrt{3}}</math><br />
 +
 
 +
 
 +
 
 +
:      <math>f_a(x_1)=\;</math><br />
 +
: <math>= f_a(1 + \sqrt{3})\;</math>
 +
: <math>= ( 1 + \sqrt{3} - a )\cdot e^{a + 2 - ( 1 + \sqrt{3})}</math><br />
 +
:                      <math> = ( 1 + \sqrt{3} - 2 )\cdot e^{2 + 2 - ( 1 + \sqrt{3})}</math><br />
 +
:                      <math> = ( \sqrt{3} - 1 )\cdot e^{4 - 1 - \sqrt{3})}</math><br />
 +
:                      <math> = ( \sqrt{3} - 1 )\cdot e^{3 - \sqrt{3})}</math><br />
 +
:                      <math>\approx 2{,}601</math><br />
 +
 
 +
 
 +
:                      <math> \Rightarrow B_1(1 + \sqrt{3} / 2{,}601)</math>
 +
 
 +
 
 +
 
 +
:      <math>f_a(x_2) =\;</math><br />
 +
:      <math>= f_a(1 - \sqrt{3})\;</math>
 +
:                      <math> = ( 1 - \sqrt{3} - a )\cdot e^{a + 2 - ( 1 - \sqrt{3})}</math><br />
 +
:                      <math> = ( 1 - \sqrt{3} - 2 )\cdot e^{2 + 2 - ( 1 - \sqrt{3})}</math><br />
 +
:                      <math> = ( -\sqrt{3} - 1 )\cdot e^{4 - 1 + \sqrt{3})}</math><br />
 +
:                      <math> = ( -\sqrt{3} - 1 )\cdot e^{3 + \sqrt{3})}</math><br />
 +
:                      <math>\approx -310{,}164</math><br />
 +
 
 +
 
 +
:                      <math> \Rightarrow B_2(1 - \sqrt{3} / -310{,}164)</math>
 +
 
 +
 
 +
 
 +
<ggb_applet width="615" height="522"  version="3.2" ggbBase64="UEsDBBQACAAIALYJODwAAAAAAAAAAAAAAAAMAAAAZ2VvZ2VicmEueG1s3VdLk9s2DD43v4Kj0247tvWw/JixN9Okl8xs2oOTHHJohpJgm12JVEVqV95fXxCkZDvbTHe3yXSag0cUAILAhw+gvHrZVSW7hUYLJddBNA4DBjJXhZC7ddCa7WgRvLx6sdqB2kHWcLZVTcXNOkjGcWDlrbh68cNK79Ud4yWZfBBwtw5M00LAdN0AL/QewDjxlpca5bztRCl4c/gt+wNyo48K5+ONrFvTO8mr4lro/nVC59WlML+IW1FAw0qVr4NZipHj6gM0RuS8XAfT0EnidRCH0zMlihKr3atG3CtprPnR+RYljGlxDwhIbGWrCeW5gjYvRSG4tMlQHGjE2J0ozN4emKJLELs9xprGofOWK9UUm4M2ULHuIzSKwhnPl0mUztJoGS+Xs1nADk6VzMfzRTqfzmfxPFmmyQIxxIAxksU4SadoukzDKQa/nOMer4pQFScp7kGLZbKYpe5ouN2AMVhJzXgHusdz14hiANy+vNGvVHkU1UpI85rXpm2IBYkXbczBnoa4NTbHn+WuBC+LsUp7yG8y1W0cbolz/e5Q0xaKJ9u9VqVqWIMbUsRq55+Ze5KNDXSwCskmJAvvwzod9IgeWdAzc0+yKoV0ofnEoz7rKOyPEZpZATq35O2xKXkGSIaAtVKY6/4FSXPjM42c/a9tlWHTnNJmcBl9JZeryWd8W91AI6F0rJJY2Fa1mt1a9rrSURwF5KLCV6fwgHBbrPcYgJMWsGugj9u1nIOLtGfM/Uy8mvRB2Bg0xpobHB2Yj7G52NY22FZ2VXBjJbZvSqgAm8oQG2RbQSPyARke2NPwiNYfFI99BDRWFI2IgZ1+0xF31H+BMDhN6j3H1TjytOAHnBenOZK3t6rwJ3s7XdJgqQSOxFFqZ2LFO+xpu+KZVmVrYJMjgvJa5dzQ6HTF9qMgCkNr29k5YxcH3EyLrejg2Gl/P3+O5DV7ZIkEranDzGkvcYlFJuRxstQuRxy3AI6AvS2rMWfq4oEhSCtXjn8sDDwszDxaxAv7m6bLcJr+t2UiMJ3kpA37q+VccZZ1h9eStjdeH+b2E8d7r6tpddFdsjW76NiI8Uv2I4PfL5CR7CfGUdJdBmzyALltK6kNglOHj67lGX5nQ+Np8IWPhC98NM++zBW6EYY4X32Kg3+bCV0Fz83l+e3g55y2zToKx/OEunWUROgC7+V791FEhu4WtFc+HZ08DaHoe0Ao7gGKx7Mw+nrwvP8ewOlHPT2fgkyuqorLgkle4TnX2JcEh7BfwYyHFh7GI0ciB0JrelXmnHkXD2C2TT6gmH27mdR/0KURVcM+nluMk370LDv0xLs/dfeYNOBP6Wy0+9YSFf5zyIV5fgXiBxUonlCB4n9WgX4MHobh+M1KMDn9nqT/XP4/59VfUEsHCBNBW6gUBAAApQ4AAFBLAQIUABQACAAIALYJODwTQVuoFAQAAKUOAAAMAAAAAAAAAAAAAAAAAAAAAABnZW9nZWJyYS54bWxQSwUGAAAAAAEAAQA6AAAATgQAAAAA" framePossible = "true" showResetIcon = "false" showAnimationButton = "true" enableRightClick = "false" errorDialogsActive = "true" enableLabelDrags = "false" showMenuBar = "false" showToolBar = "false" showToolBarHelp = "false" showAlgebraInput = "false" /><br />
 +
<br />
 +
 
 +
<ggb_applet width="595" height="433"  version="3.2" ggbBase64="UEsDBBQACAAIAM0IODwAAAAAAAAAAAAAAAAMAAAAZ2VvZ2VicmEueG1s3VdLj9s2ED43v4LgabeFbVIP2wvYGzTpJcCmPTibQw4NKGlssyuRqkjtyvvrOyQlP7INuk5TtA0MQ9TMaDjzzTdDafGyq0pyD42RWi0pHzNKQOW6kGqzpK1dj+b05fWLxQb0BrJGkLVuKmGXNB5H1Mlbef3iu4XZ6gciSm/yXsLDktqmBUpM3YAozBbABvFalAblou1kKUWz+yX7DXJrDorg442qWzs4yaviRprhduL3q0tpf5L3soCGlDpf0mmKkePqPTRW5qJc0oQFSbSkEUtOlCiKnXarG/molXXmB+drlBBi5CMgIJGTLSY+zwW0eSkLKZRLxseBRoQ8yMJu0eU8RZcgN1uMNYl58JZr3RSrnbFQke4DNHpJ5+l4xtIp41HE2TROrijZBU2UJuOUzWb8apryK57EqDIYMEYSx2OUTqdJxFOWcM7xoYNqOmcpT6KYxVP8hZ3hfgXWYiENER2YAc5NI4s93u7mjXmly4Oo1lLZ16K2beNJEPeild25zRC2xqX4o9qU0MsiLNIW8rtMd6sAWxxcv9vV/hEfT7Z5rUvdkMbliVBt+msWrt7GBbq3Yt6GeYveh3O61/OryFv4axau3qqUKoTWJ86HrDkbtpGGOAE6d9wdsClFBsgFSlol7c1wg5y56zPlwf7ntsqwZ45Zs3fJv5LLxeQTui3uoFFQBlIpLGyrW0PuHXlD6XwcBeSywtug6AERrli3GECQFrBpYIg7dFyAy2vZMXE/ES8mQxAuBoOx5hYnB+ZjXS6usy12lVsVwjqJa5sSKsCesp4Nqq2gkfkeGUHdbrhF228UjfsI/FTRfkLs2dk/dMAd9Z8hDA6TeitwNe5bsRQ7HBfHOXpvb3XR79zbmdLPlUriRBylbiRWoltSvxKZ0WVrYZUjgupG58L6yRmK3U8Czpiz7dyYcYsdPuwXa9nBodP+fPwcyGu3yBIFxvgOs8e9JBQW2SOPg6UOOeK0BQgEHGxJjTn7Lt4zBGkVyvGXhYGnhZnxeTR3/yS9Ykn675bJgxkkR204nCynipOsOzyVjDvwhjDXHwUee13tVxfdJVmSi46MiLgk3xP49QIZSX4gAiXdJSWTJ8itW+XbgB47fHYtT/A7GRrnwceeCR97Ns8+zxV/IuzjfPUxon83E38UfGkuX94O/ZwzrllHbDyLfbeOYo4uppQ8hncibxhOQXfi+63j8xDi3wJC0QBQNMbXl68Hz+23AM4w6v31HGRyXVVCFUSJCve5wb70cEj3EkwEc/AQwQOJAgitHVRZcNa7eAKza/I9itl/fiYd9WLPsN1Ausdjd89JAX5XwcaE9yxZ4UdDLu3Z6Lvp5vG/fYJ+fgb6+f8J/WH+eTr/o/BPjt8j/adW/6l5/QdQSwcIg1ollA0EAACcDgAAUEsBAhQAFAAIAAgAzQg4PINaJZQNBAAAnA4AAAwAAAAAAAAAAAAAAAAAAAAAAGdlb2dlYnJhLnhtbFBLBQYAAAAAAQABADoAAABHBAAAAAA=" framePossible = "true" showResetIcon = "false" showAnimationButton = "true" enableRightClick = "false" errorDialogsActive = "true" enableLabelDrags = "false" showMenuBar = "false" showToolBar = "false" showToolBarHelp = "false" showAlgebraInput = "false" />
 +
 
 +
<ggb_applet width="615" height="430"  version="3.2" ggbBase64="UEsDBBQACAAIAMwKODwAAAAAAAAAAAAAAAAMAAAAZ2VvZ2VicmEueG1s3VdLj9s2ED43v4LQabeFbeotAfYGTXoJsGkPbnLooQEl0Ta7EqmK1K7sX98hKcmv3TTeGmjRgyFqOBzOfPPNjDx/21UleqSNZIIvHHeKHUR5LgrG1wunVatJ4ry9ezNfU7GmWUPQSjQVUQvHn3qOlrfs7s13c7kRT4iURuUzo08LRzUtdZCsG0oKuaFUWfGKlBLkpO1YyUiz/SX7g+ZK7jesjQ+8btVgJK+KeyaH15m5ry6Z+ok9soI2qBT5wolC8BxWn2mjWE7KhRNgK/EWjoeDo00Q+Xp3Ixq2E1xp9b3xFUgQkmxHARBPy+YzE+ectnnJCka4Dsb4AUoIPbFCbfSFIZikbL0BXwMvsdZyIZpiuZWKVqj7jTYCjLrJNI39NMJpFMbm2NbuBHjqJm4SxXEceGkQB+C3BIfBEw/OYBz5Qeh5aRrDEk71e3iapGnip26c4Ah0kv5u+rikSkEqJSIdlQOg64YVI+L65YN8J8q9qBaMq/ekVm1jaOD3oqXamtsc1Oggf+TrkvYyD9K0oflDJrqlBc63pn/d1uaI8SdbvxelaFADB0KIet0/M/s0OtrRUQsbHWw0ehva6Ljvpp7RMM/MPo1Wybh1rQ/cHaJ28XANk0gLwLhm74BNSTIKbHBQy5m6H16ANQ99pK7V/7mtMqiaQ96MJt0rmZzPTgg3f6ANp6WlFYfEtqKV6FHT16bO+FHQnFXwajd6QIhO1idwwEoLum7o4LetOQuX2cWH1D0Rz2eDE9oHCb7mCnoHxKN0LLq2FdSVXhVEaYkunJJWFKpKGTbwtqINy0dkiKNvgyva/iJv2ntg+oowPWJkZ39ojzvsv0AYaCf1huj6cHtakC00jMMYjbWPouhv7vVkaTpLxaAnTkLdFCvSLRyzIpkUZavoMgcE+b3IiTK90ya77wUuxlq3041GL7Zw2CxWrKP7Snu+Ae3JqzbAEk6lNBWmDmuJcEiyQR5aS21jhH5LqSXgoItqiNlU8cgQoJVNx98mhp4nJnYTL9G/IExxEP67aTJgWslBGQ6z5XjjKOoO5pLUI29wc/WFwODrarO66W7RAt10aILILfoe0d9vgJHoB0RA0t06aHaG3KrlpgycQ4Mv5tI/yeURfkdN4zL48DfCh7/Cs2PfXuaKmQijn+++eM4/jcSMgtfG8vpy6Puc1MU6wdPYN9U68V0wATN2Z7+KjKKdgnrmm6v9yxByzxG6tFb+AxAF0yBIgySK/BDHfpRatKA+oOX5PvbjKE5CPwmvh9yn/wOzhilgnpcgk4uqIrxAnFRwzz2UrIGD6S9kRLCGBxHX8suC0KphK7PGehNnMOv6H1HMXm5Xp6Pnau3+wkzs0XyObtvnuLkzNf21fncaHP2TWx1pP85YBf81cqZenxfvLC/FBXkpXpmXbyiR4RvcJiZ0r5GZoW1ux2a6O7R3zQzMDr8/zZ+0/k/q3V9QSwcIhWpWpUMEAADWDgAAUEsBAhQAFAAIAAgAzAo4PIVqVqVDBAAA1g4AAAwAAAAAAAAAAAAAAAAAAAAAAGdlb2dlYnJhLnhtbFBLBQYAAAAAAQABADoAAAB9BAAAAAA=" framePossible = "true" showResetIcon = "false" showAnimationButton = "true" enableRightClick = "false" errorDialogsActive = "true" enableLabelDrags = "false" showMenuBar = "false" showToolBar = "false" showToolBarHelp = "false" showAlgebraInput = "false" />

Aktuelle Version vom 24. Januar 2010, 02:23 Uhr

Inhaltsverzeichnis

Tangente im Punkt Wa( a + 2 / 2 ) an Gfa mit dem Schnittpunkt A (0 / 2012 )

mit:\;

x = 0\;
y = 2012\;
x_0 = a + 2\;
f_a( x_0 ) = f_a( a + 2 ) = 2\;
f^{'}_a( x_0 ) = f^{'}_a( a + 2 ) = m = -1\;


f^{'}_a( a + 2 ) = e^{a + 2 - ( a + 2 )}\cdot ( 1 + a - ( a + 2 ) )
= e^{a + 2 - a - 2 }\cdot ( 1 + a - a - 2 ) )
= e^{0}\cdot ( -1 ) )
= -1\;


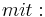
Lösung; Tangentengleichung

Tangentengleichung: siehe Formelsammlung Seite 58

y = f^{'}( x_0 )\cdot ( x - x_0 ) + f ( x_0)


y = f^{'}_a( a + 2 )\cdot ( x - ( a + 2 )) + f ( a + 2 )
y = (-1)\cdot ( x - a - 2 ) + 2
y = -x + a + 2 + 2\;
y = -x + a + 4\;
2012 = 0 + a + 4\;\;\;\;\;\;\; | -4
a = 2008\;

Lösung; Fußweg

y = m\cdot x + t
f_a( x_0 ) = f^{'}_a( x_0 )\cdot x_0 + t
f_a( a + 2 ) = f^{'}_a( a + 2 )\cdot x_0 + t
2 = -1\cdot x_1 + t \;\;\;\;\;\; | - ( -1\cdot x_0)
t = 2 - ( -1\cdot x_0 )
t = 2 - ( -1\cdot ( a + 2 ))
t = 2 - ( -a - 2)\;
t = 2 + a + 2 \;
t = a + 4 \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\; |\;einsetzen\; in\; y = m\cdot x + t


y = m\cdot x + a + 4
2012 = -1\cdot 0 + a + 4
2012 = a + 4 \;
a = 2008\;

Lösung; Clever

\frac{y_2 - y_1}{x_2 - x_1} = f{'}_a ( x )
\frac{2012 - 2}{0 - ( a + 2 )} = -1
\frac{2010}{(-a - 2 )} = -1 \;\;\;\;\;\;\;\;\;\;| \cdot( -a - 2 )
2010 = a + 2\;
2008 = a\;

Berechnung derjenigen Punkte, für welche die Tangente an den Graphen von f2 durch den Ursprung verläuft

Verwendung der Tangentialgleichung

y = f^{'}( x_0)\cdot ( x - x_0 ) + f ( x_0 )
y = ( x_0 - a - 1 )\cdot ( -e^{a + 2 - x_0})\cdot ( x - x_0 ) + ( x_0 - a )\cdot e^{a + 2 - x_0})


mit:\;

y = 0\;
x = 0\;
a = 2\;


0 = ( x_0 - 3 )\cdot ( -e^{4 - x_0} )\cdot ( -x_0 ) + ( x_0 - 2 )\cdot ( e^{4 - x_0} )
0 = ( x_0 - 3 )\cdot ( e^{4 - x_0} )\cdot ( x_0 ) + ( x_0 - 2 )\cdot ( e^{4 - x_0} )
0 = ( x_0^{2} - x_0\cdot 3 )\cdot ( e^{4 - x_0} ) + ( x_0 - 2 )\cdot ( e^{4 - x_0} )
0 = e^{4 - x_0}\cdot ( x_0^{2} - 3\cdot x_0 + x_0 - 2 )
0 = e^{4 - x_0}\cdot ( x_0^{2} - 2\cdot x_0 - 2 )\;\;\;\;\;\;\;\;|e^{4 - x_0}>0
\Rightarrow ( x_0^{2} - 2\cdot x_0 - 2 ) = 0

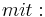
Lösen quadratischer Gleichungen mit Hilfe der Mitternachtsformel x_{1,2} = \frac{-b\pm\sqrt{b^{2}-4\cdot a\cdot c}}{2a}

x_{1,2} = \frac{2\pm\sqrt{4--8}}{2}
x_{1,2} = \frac{2\pm\sqrt{4+8}}{2}
x_{1,2} = \frac{2\pm\sqrt{12}}{2}
x_{1,2} = \frac{2\pm\sqrt{4\cdot 3}}{2}
x_{1,2} = \frac{2\pm2\cdot\sqrt{3}}{2}
x_{1,2} = {1\pm\sqrt{3}}


\Rightarrow x_{1} = {1 + \sqrt{3}}
\Rightarrow x_{2} = {1 - \sqrt{3}}


f_a(x_1)=\;
= f_a(1 + \sqrt{3})\;
= ( 1 + \sqrt{3} - a )\cdot e^{a + 2 - ( 1 + \sqrt{3})}
= ( 1 + \sqrt{3} - 2 )\cdot e^{2 + 2 - ( 1 + \sqrt{3})}
= ( \sqrt{3} - 1 )\cdot e^{4 - 1 - \sqrt{3})}
= ( \sqrt{3} - 1 )\cdot e^{3 - \sqrt{3})}
\approx 2{,}601


\Rightarrow B_1(1 + \sqrt{3} / 2{,}601)


f_a(x_2) =\;
= f_a(1 - \sqrt{3})\;
= ( 1 - \sqrt{3} - a )\cdot e^{a + 2 - ( 1 - \sqrt{3})}
= ( 1 - \sqrt{3} - 2 )\cdot e^{2 + 2 - ( 1 - \sqrt{3})}
= ( -\sqrt{3} - 1 )\cdot e^{4 - 1 + \sqrt{3})}
= ( -\sqrt{3} - 1 )\cdot e^{3 + \sqrt{3})}
\approx -310{,}164


\Rightarrow B_2(1 - \sqrt{3} / -310{,}164)




Von „http://rmg.zum.de/index.php?title=Facharbeit_Andre_Etzel/Teilaufgabe_c/Lösung_von_Teilaufgabe_c&oldid=27803“