2006 V: Unterschied zwischen den Versionen

Aus RMG-Wiki
Wechseln zu: Navigation, Suche
Zeile 24: Zeile 24:
 
<tr><td  width="800px" valign="top">
 
<tr><td  width="800px" valign="top">
  
−
;Aufgabe 1
 
 
In einem kartesischen Koordinatensystem des <math>\mathbb{R} </math><sup>3</sup> ist die  
 
In einem kartesischen Koordinatensystem des <math>\mathbb{R} </math><sup>3</sup> ist die  
 
Ebene E: x<sub>2</sub> - x<sub>3</sub> - 1 = 0 , die Geradenschar g<sub>k</sub> : <math>\vec x = \begin{pmatrix} -k^2 \\ 0 \\ -1 \end{pmatrix} + \lambda \cdot\begin{pmatrix} 3 \\ 2 \\ 2 \end{pmatrix}</math> und die Gerade h : <math>\vec x = \begin{pmatrix} 2 \\ 1 \\ 2 \end{pmatrix} + \mu \cdot\begin{pmatrix} 0 \\ -1 \\ 2 \end{pmatrix}</math> gegeben, wobei k, <math>\lambda</math> und <math>\mu</math>  aus <math>\mathbb{R} </math> sind.
 
Ebene E: x<sub>2</sub> - x<sub>3</sub> - 1 = 0 , die Geradenschar g<sub>k</sub> : <math>\vec x = \begin{pmatrix} -k^2 \\ 0 \\ -1 \end{pmatrix} + \lambda \cdot\begin{pmatrix} 3 \\ 2 \\ 2 \end{pmatrix}</math> und die Gerade h : <math>\vec x = \begin{pmatrix} 2 \\ 1 \\ 2 \end{pmatrix} + \mu \cdot\begin{pmatrix} 0 \\ -1 \\ 2 \end{pmatrix}</math> gegeben, wobei k, <math>\lambda</math> und <math>\mu</math>  aus <math>\mathbb{R} </math> sind.
  
 +
 +
<div style="padding:1px;background: #EEEEE6;border:0px groove;">
 +
 +
 +
<center><table border="0" width="800px" cellpadding=5 cellspacing=15>
 +
<tr><td  width="800px" valign="top">
 +
 +
;Aufgabe 1
  
 
a) Zeigen Sie: Alle Geraden der Schar g<sub>k</sub> sind zueinander parallel und liegen in der Ebene E.  
 
a) Zeigen Sie: Alle Geraden der Schar g<sub>k</sub> sind zueinander parallel und liegen in der Ebene E.  

Version vom 25. Februar 2010, 14:46 Uhr


Leistungskurs Mathematik (Bayern): Abiturprüfung 2006
Analytische Geometrie V.


Download der Originalaufgaben: Abitur 2008 LK Mathematik Bayern - Lösung gesamt


Erarbeitet von Johannes Brunnquell, Lea Mainberger, Maximilian Benkert


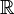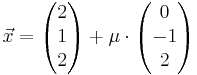In einem kartesischen Koordinatensystem des \mathbb{R} 3 ist die Ebene E: x2 - x3 - 1 = 0 , die Geradenschar gk : \vec x = \begin{pmatrix} -k^2 \\ 0 \\ -1 \end{pmatrix} + \lambda \cdot\begin{pmatrix} 3 \\ 2 \\ 2 \end{pmatrix} und die Gerade h : \vec x = \begin{pmatrix} 2 \\ 1 \\ 2 \end{pmatrix} + \mu \cdot\begin{pmatrix} 0 \\ -1 \\ 2 \end{pmatrix} gegeben, wobei k, \lambda und \mu aus \mathbb{R} sind.



Aufgabe 1

a) Zeigen Sie: Alle Geraden der Schar gk sind zueinander parallel und liegen in der Ebene E.

3 BE


.


b) Begründen Sie, dass die Schar der Geraden gk eine Halbebene von E bildet.

4 BE


.


c) Für welche Werte von k schneidet gk die Gerade h? Ermitteln Sie die Koordinaten des Schnittpunkts S.

[ Teilergebnis: S = (2/\frac{5}{3}/\frac{2}{3}) ]

5 BE


.


d) Projiziert man h senkrecht auf E, so erhält man die Gerade hE. Berechnen Sie den Winkel \varphi zwischen hE und h in Grad auf eine Nachkommastelle gerundet.

5 BE


.


Aufgabe 2

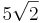Die Ebene E ist Tangentialebene an zwei Kukeln K1 und K2 mit dem Radius 5\sqrt{2}, deren Mittelpunkte M1 und M2 auf der Gerade h liegen.


a) Bestimmen Sie die Koordinaten von M1 und M2 . (Der Punkt mit ausschließlich ganzzahligen Koordinaten wird mit M1 bezeichnet.)

[Teilergebnis: (2/5/-6)]

6 BE


.


b) Die Kugelpunkte P \in K1 und Q \in K2 sind diejenigen Punkte, die minimale Distanz voneinander haben. Berechnen Sie die Entfernung [PQ] auf zwei Dezimalen gerundet.

3 BE


.


c) Spiegelt man die Ebene E am Punkt M1, so erhält man die Ebene*. Geben Sie eine Gleichung von E* in Normalenform an.

4 BE


.


d) Zeigen Sie, dass die Punkte A (-1/0/-2) und C (-1/1/-1) auf der Kugel K1 um M1 liegen und bestimmen Sie die Koordinaten von B so, dass die Strecke [AB] ein Durchmesser von K1 ist.

[Teilergebnis: B (5/10/-10)]

4 BE


.


e) Das Dreieck ABC ist die Grundfläche einer Pyramide ABCD, deren Spitze D ebenfalls auf der Kugel K1 liegt. Alle Punkte D, für die die Pyramiden ABCD das Volumen 11 haben, bilden zwei Kreise auf der Kugelfläche (Nachweis nicht erforderlich). Berechnen Sie zuerst die Höhe h dieser Pyramiden und anschließend mit Hilfe einer geeigneten Skizze den Radius R der beiden oben definierten Kreise.

[Zur Kontrolle: h = \sqrt{11}]

6 BE


.



Fehler beim Parsen(Syntaxfehler): \left( -k

Von „http://rmg.zum.de/index.php?title=LK_Mathematik/Abitur/2006_V&oldid=30149“