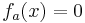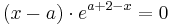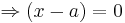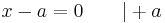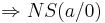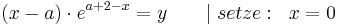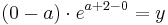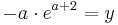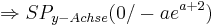Lösung: Schnittpunkte mit den Koordinatenachsen: Unterschied zwischen den Versionen
Aus RMG-Wiki
(→2. Schnittpunkt mit der y-Achse) |
|||
| Zeile 7: | Zeile 7: | ||
====1. Nullstellen ==== | ====1. Nullstellen ==== | ||
| + | |||
:<math>f_a (x) = 0\;</math><br /> | :<math>f_a (x) = 0\;</math><br /> | ||
| Zeile 27: | Zeile 28: | ||
Für <math>a > 0\;</math> folgt: <math>\;\;\;\; NS ( >0 / 0 )\;</math><br /> | Für <math>a > 0\;</math> folgt: <math>\;\;\;\; NS ( >0 / 0 )\;</math><br /> | ||
Für <math>a = 0\;</math> folgt: <math>\;\;\;\; NS ( 0 / 0 )\;</math><br /> | Für <math>a = 0\;</math> folgt: <math>\;\;\;\; NS ( 0 / 0 )\;</math><br /> | ||
| + | |||
====2. Schnittpunkt mit der y-Achse ==== | ====2. Schnittpunkt mit der y-Achse ==== | ||
| + | |||
:<math>( x - a )\cdot e^{a+2-x} = y \;\;\;\;\;\;\; |\; setze:\;\; x = 0</math> <br /> | :<math>( x - a )\cdot e^{a+2-x} = y \;\;\;\;\;\;\; |\; setze:\;\; x = 0</math> <br /> | ||
Version vom 23. Januar 2010, 00:24 Uhr
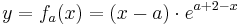 mit
mit 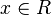 ;
; 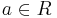
Schnittpunkte mit den Koordinatenachsen
1. Nullstellen
Da die e-Funktion ( in diesem Fall ea + 2 - x) immer streng monoton steigend und
immer positiv ist, gibt es nur für ( x - a ) = 0 Nullstellen.
Für 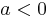 folgt:
folgt: 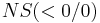
Für 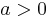 folgt:
folgt: 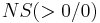
Für 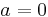 folgt:
folgt: 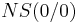
2. Schnittpunkt mit der y-Achse
Für 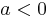 folgt:
folgt: 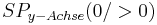
Für 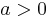 folgt:
folgt: 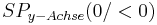
Für 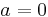 folgt:
folgt: