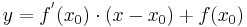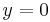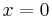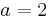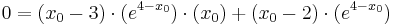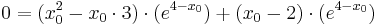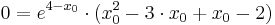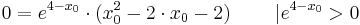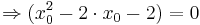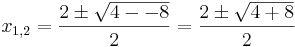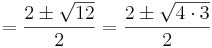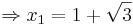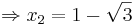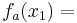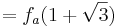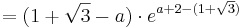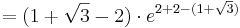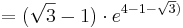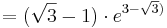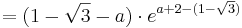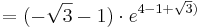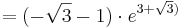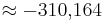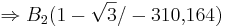Lösung von Teilaufgabe c) 2.
Aus RMG-Wiki
< Facharbeit Andre Etzel | Teilaufgabe c
Version vom 26. Januar 2010, 19:46 Uhr von Andre Etzel (Diskussion | Beiträge)
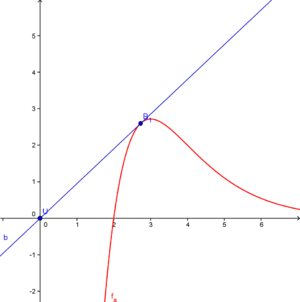
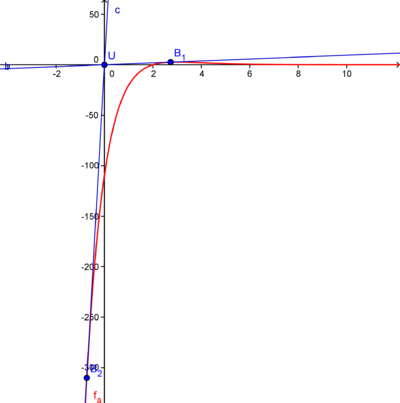
Berechnung derjenigen Punkte, für welche die Tangente an den Graphen von f2 durch den Ursprung verläuft
Verwendung der Tangentengleichung
Hier rate ich wieder zur Verwendung der Tangentengleichung.
Zwar fehlen hier einige feste Werte die man in die Gleichung einsetzen könnte, dochdiese hat man in allgemeiner Form durch die Funktion und die Ableitung gegeben.
Lösen quadratischer Gleichungen mit Hilfe der Mitternachtsformel 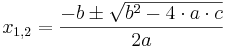
Fehler beim Erstellen des Vorschaubildes: Ungültige Thumbnail-Parameter