Lösungsübersicht: Unterschied zwischen den Versionen
Aus RMG-Wiki
(Die Seite wurde neu angelegt: == Funktion == Stammfunktion: <math> F_a (x) = ( x - a + 1 )\cdot (-e^{a + 2 - x}) </math> <br /> Funktion: <math> y = f_a (x) = ( x - a )\cdot e^{a + 2 - x}...) |
(→Teilaufgabe b)) |
||
| Zeile 18: | Zeile 18: | ||
=== Teilaufgabe b) === | === Teilaufgabe b) === | ||
| − | 1. | + | 1. <math>-\infty < x < a </math> G<sub>F<sub>a</sub></sub> str. mon. fallend |
| + | <math>a < x < \infty </math> G<sub>F<sub>a</sub></sub> str. mon. steigend | ||
| + | <math>x = a</math> hat G<sub>F<sub>a</sub></sub> eine Tiefpunkt | ||
2. Stammfunktion: <math> F_a (x) = ( x - a + 1 )\cdot (-e^{a + 2 - x}) </math> <br /> | 2. Stammfunktion: <math> F_a (x) = ( x - a + 1 )\cdot (-e^{a + 2 - x}) </math> <br /> | ||
3. Flächeninhalt zw. x-Achse und f<sub>2</sub>: A = e<sup>2</sup> | 3. Flächeninhalt zw. x-Achse und f<sub>2</sub>: A = e<sup>2</sup> | ||
| − | |||
=== Teilaufgabe c) === | === Teilaufgabe c) === | ||
Version vom 5. Januar 2010, 02:16 Uhr
Inhaltsverzeichnis |
Funktion
Stammfunktion: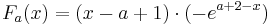
Funktion: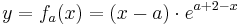
1. Ableitung:
2. Ableitung: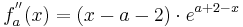
3. Ableitung: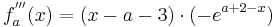
Teilaufgabe a)
Nullstelle: NS ( a / 0 ) Schnittpunkt mit der y-Achse: SPy-Achse ( 0 / -a * ea + 2) Extrempunkt: Max ( 1 + a / e ) Wendepunkt: WP ( a + 2 / 2 ) Funktionsgleichung aller Extrempunkte: h (x) = e
Teilaufgabe b)
1.GFa str. mon. fallend
GFa str. mon. steigend
hat GFa eine Tiefpunkt 2. Stammfunktion:
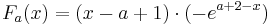
3. Flächeninhalt zw. x-Achse und f2: A = e2
Teilaufgabe c)
1. a = 2008 2.