Lösung von Teilaufgabe b: Unterschied zwischen den Versionen
(→3. Flächenberechnung, der sich nach rechts ins Unendliche erstreckenden Fläche, zwischen der x- Achse und der Funktion f2 im I. Quadranten) |
K (Schützte „Facharbeit Andre Etzel/Teilaufgabe b/Lösung von Teilaufgabe b“: f [edit=sysop:move=sysop] [kaskadierend]) |
||
| (22 dazwischenliegende Versionen von einem Benutzer werden nicht angezeigt) | |||
| Zeile 1: | Zeile 1: | ||
=== 1. Eigenschaften einer Stammfunktion von f<sub>a</sub> === | === 1. Eigenschaften einer Stammfunktion von f<sub>a</sub> === | ||
| − | 1.) Von <math>-\infty < x < a </math> verläuft der Graph G<sub>f<sub>a</sub></sub> unterhalb der x-Achse und ist somit | + | 1.) Von <math>-\infty < x < a </math> verläuft der Graph G<sub>f<sub>a</sub></sub> unterhalb der x-Achse und ist somit negativ. Daraus kann man schließen, dass der Graph G<sub>F<sub>a</sub></sub> in diesem Intervall streng monoton fallend ist.<br /> |
| − | Von <math>a < x < \infty </math> verläuft der Graph G<sub>f<sub>a</sub></sub> oberhalb der x-Achse und ist somit | + | Von <math>a < x < \infty </math> verläuft der Graph G<sub>f<sub>a</sub></sub> oberhalb der x-Achse und ist somit positiv. Daraus kann man schließen, dass der Graph G<sub>F<sub>a</sub></sub> in diesem Intervall streng monoton steigend ist.<br /> |
| − | 2.) Bei <math>x = a</math> ist der Graph G<sub>f<sub>a</sub></sub> gleich Null ( G<sub>f<sub>a</sub></sub> = 0 )und das | + | 2.) Bei <math>x = a\,</math> ist der Graph G<sub>f<sub>a</sub></sub> gleich Null ( G<sub>f<sub>a</sub></sub> = 0 )und das Steigungsverhalten von G<sub>F<sub>a</sub></sub> ändert für <math>x < a</math> und <math>x > a</math> das Vorzeichen. Deshalb kann man sagen, dass der Graph G<sub>F<sub>a</sub></sub> an der Stelle <math>x = a</math> einen Extrempunkt, in diesem Fall einen Tiefpunkt ( Minimum ) hat, da sich das Monotonieverhalten von streng monoton fallend nach streng monoton steigend ändert. |
=== 2. Bestimmung einer Stammfunktion von f<sub>a</sub> durch partielle Integration === | === 2. Bestimmung einer Stammfunktion von f<sub>a</sub> durch partielle Integration === | ||
[http://de.wikipedia.org/wiki/Partielle_Integration Hilfe zur partiellen Integration] | [http://de.wikipedia.org/wiki/Partielle_Integration Hilfe zur partiellen Integration] | ||
| + | |||
| + | <math> \int_a^b f(x)\cdot g'(x)\,\mathrm{d}x | ||
| + | = [f(x)\cdot g(x)]_{a}^{b} - \int_a^b f'(x)\cdot g(x)\,\mathrm{d}x.</math> | ||
| + | |||
<math> \int_{a}^{b} f_a ( x )\,dx = ( x - a ) e^{a + 2 - x}</math> | <math> \int_{a}^{b} f_a ( x )\,dx = ( x - a ) e^{a + 2 - x}</math> | ||
| Zeile 20: | Zeile 24: | ||
<math>v ^{'} ( x ) = -e^{a + 2 - x}</math> | <math>v ^{'} ( x ) = -e^{a + 2 - x}</math> | ||
| − | <math> \int_{a}^{b} f_a ( x )\,dx = ( x - a ) e^{a + 2 - x}</math> <br /> | + | <math> \int_{a}^{b} f_a ( x )\,dx = ( x - a )\cdot e^{a + 2 - x}</math> <br /> |
::: <math>=[( x - a )\cdot (-e^{a + 2 - x}) ]^{b}_{a} - \int_{a}^{b} 1 \cdot (-e^{a + 2 - x})\,dx</math> | ::: <math>=[( x - a )\cdot (-e^{a + 2 - x}) ]^{b}_{a} - \int_{a}^{b} 1 \cdot (-e^{a + 2 - x})\,dx</math> | ||
::: <math>=[( x - a )\cdot (-e^{a + 2 - x}) - e^{a + 2 - x}]^{b}_{a}</math> | ::: <math>=[( x - a )\cdot (-e^{a + 2 - x}) - e^{a + 2 - x}]^{b}_{a}</math> | ||
| − | ::: <math>=[-e^{a + 2 - x} ( x - a + 1 )]^{b}_{a}</math> | + | ::: <math>=[-e^{a + 2 - x}\cdot ( x - a + 1 )]^{b}_{a}</math> |
:::: <math>\Rightarrow F_a( x ) = -e^{a + 2 - x} ( x - a + 1 ) + c</math><br /> | :::: <math>\Rightarrow F_a( x ) = -e^{a + 2 - x} ( x - a + 1 ) + c</math><br /> | ||
| + | für Interessierte: [[Facharbeit Andre Etzel/Teilaufgabe b/Lösung von Teilaufgabe b/Der Holzweg|Der Holzweg]] | ||
| − | |||
| − | |||
| − | + | <ggb_applet width="671" height="418" version="3.2" ggbBase64="UEsDBBQACAAIAJoUOjwAAAAAAAAAAAAAAAAMAAAAZ2VvZ2VicmEueG1svVZZj9s2EH5ufgWhp02DtUUdPgBrgyZFgQCbA3Cbhz6koKSxzS5FCiS1K+fXZ0hKtuwmbQoUfTA0nBnO8c1Bb172jSCPoA1XsojoLI4IyErVXO6LqLO721X08u7ZZg9qD6VmZKd0w2wRpbMkcvyO3z37YWMO6okw4VU+cngqoh0TBiJiWg2sNgcAe8FnXc8FZ/r4vvwTKmvOgmDkjWw79GJ1h7yqqe+5GY9z77AV3P7MH3kNmghVFdEix9CR+gja8oqJIsriwEmKKLkSIit10oPS/LOS1qmfje+QQ4jhnwERSQKvUtJ80Mq+VqJrpCGkUiI+BagEndDJhE5PmeEhmwjyUXBhXqGEdAbQjdJmVHfwvpfi+ArBfGgVl2fA8PZm7quwga4SvOZMOqQ9SJgBIU+8tgfEZ0kxX+D7AwKZ0cXoV+l6ezQWGtL/DlohVhl2QbpMl8s1pWmWrfOIHIOIrrNZTOkiy9PlOqOpExmEE3FKl7NFnKU5XSXrZLFKV3hpECV4KVmtUrrK0yxeL/LBNzxuwVpsNENYD6dk95rXJ9Dc4Y15pcSZ5fN/zVrbad+k6cDa2qPzhlXVLsmf5F7AwEsQ8QNUD6Xqt6GqaTD967H1V3w85d6jTrTrF8xsP3zL8PU6LtCTVux1Yq8x2HBGT3K6TryG/5bh67UElyG0IXE6Zk3j0Q03xDHiUPwRG8FKwFaNSCe5vR8P2NIPQ6Y06L/rmhJO/XNpkv5HJjfzq4bbPICWIIZ2xsJ2qjPk0c3WpNNrqHiDxyAYAGGuWL9hAIFbw17DGHdYCAEuL42nrXvF3szHIPxIYayVxc2G+ViXi1s8FofeUTWzjuMGR0ADOPLWd4PsGtC8OiHDIucNXXSjo9kQgd96yi+wKzTPsKP4G/2Cq649MGeODl3BjrjMpil6a29VPTge9IzwW6/huLBvc7exG9YXkadYaXBBWdhWCKC8VxWzfrGH6IZVQGOXAundEnTEES97Ysd7OA/a15fjuXftAZtEgjF+wOx0lJjEGnvgcbO0IUd8C8BZ92/MqE1azNqP8WSdDfX4x8rAdWWS2RL3z8r9snwdZ/lX6jQk938UysMZOJM5HF++S8FF1j2+msa9yGOYv/zB8F3uW0/d9M9JQW7h0w0jL0gyi8ktQdaP5KZHyvEQ4ucRmf8FwF0n/ThEU7vfLGp6VdR/2+7jEg045nQCZPydQMZ/03OX4U3xm09H37+Pw7+Xuy9QSwcI+gNsBZ4DAADvCAAAUEsBAhQAFAAIAAgAmhQ6PPoDbAWeAwAA7wgAAAwAAAAAAAAAAAAAAAAAAAAAAGdlb2dlYnJhLnhtbFBLBQYAAAAAAQABADoAAADYAwAAAAA=" framePossible = "true" showResetIcon = "false" showAnimationButton = "true" enableRightClick = "false" errorDialogsActive = "true" enableLabelDrags = "false" showMenuBar = "false" showToolBar = "false" showToolBarHelp = "false" showAlgebraInput = "false" /> | |
=== 3. Flächenberechnung, der sich nach rechts ins Unendliche erstreckenden Fläche, zwischen der x- Achse und der Funktion f<sub>2</sub> im I. Quadranten === | === 3. Flächenberechnung, der sich nach rechts ins Unendliche erstreckenden Fläche, zwischen der x- Achse und der Funktion f<sub>2</sub> im I. Quadranten === | ||
| Zeile 39: | Zeile 42: | ||
Da die Nullstelle der Funktion f<sub>a</sub> bei x = a liegt, folgt daraus, dass die Nullstelle der Funktion f<sub>2</sub> bei x = 2 liegt. Das heißt, man muss von zwei bis unendlich integrieren. | Da die Nullstelle der Funktion f<sub>a</sub> bei x = a liegt, folgt daraus, dass die Nullstelle der Funktion f<sub>2</sub> bei x = 2 liegt. Das heißt, man muss von zwei bis unendlich integrieren. | ||
| − | <math>\int_{2}^{b} f_a( x ) = [-e^{2 + 2 - x\cdot | + | <math>\int_{2}^{b} f_a( x ) = [-e^{2 + 2 - x}\cdot ( x - 2 + 1 )]^{b}_{2}</math><br /> |
::: <math> = [-e^{4 - x}\cdot ( x - 1 )]^{b}_{2}</math><br /> | ::: <math> = [-e^{4 - x}\cdot ( x - 1 )]^{b}_{2}</math><br /> | ||
::: <math> = \lim_{b\to\infty} [-e^{4 - b}\cdot ( b - 1 )] - [-e^{4 - 2}\cdot ( 2 - 1 )]</math><br /> | ::: <math> = \lim_{b\to\infty} [-e^{4 - b}\cdot ( b - 1 )] - [-e^{4 - 2}\cdot ( 2 - 1 )]</math><br /> | ||
::: <math> = \lim_{b\to\infty} [-e^{4 - b}\cdot ( b - 1 )] - [-e^{2}\cdot ( 1 )]</math><br /> | ::: <math> = \lim_{b\to\infty} [-e^{4 - b}\cdot ( b - 1 )] - [-e^{2}\cdot ( 1 )]</math><br /> | ||
| − | ::::::::::::::::::: <math> | \lim_{b\to\infty} [-e^{4 - b} ( b - 1 )] \rightarrow 0| siehe Hinweis </math><br /> | + | ::::::::::::::::::: <math> | \lim_{b\to\infty} [-e^{4 - b}\cdot ( b - 1 )] \rightarrow 0| siehe Hinweis </math><br /> |
::: <math> = 0 - [-e^{2}\cdot ( 1 )]</math><br /> | ::: <math> = 0 - [-e^{2}\cdot ( 1 )]</math><br /> | ||
| − | ::: <math> = \, [e^{2}]</math><br /> | + | ::: <math> = \, [e^{2}] \approx 7.39</math><br /> |
| − | '''Der | + | '''Der Flächeninhalt, der sich nach rechts ins Unendliche erstreckt, beträgt <span style="color: red">''e<sup>2</sup>''</span>'''. |
| + | |||
| + | |||
| + | [[Bild:Integral.png|500px]] | ||
Aktuelle Version vom 30. Januar 2010, 11:19 Uhr
1. Eigenschaften einer Stammfunktion von fa
1.) Von  verläuft der Graph Gfa unterhalb der x-Achse und ist somit negativ. Daraus kann man schließen, dass der Graph GFa in diesem Intervall streng monoton fallend ist.
verläuft der Graph Gfa unterhalb der x-Achse und ist somit negativ. Daraus kann man schließen, dass der Graph GFa in diesem Intervall streng monoton fallend ist.
Von 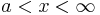 verläuft der Graph Gfa oberhalb der x-Achse und ist somit positiv. Daraus kann man schließen, dass der Graph GFa in diesem Intervall streng monoton steigend ist.
verläuft der Graph Gfa oberhalb der x-Achse und ist somit positiv. Daraus kann man schließen, dass der Graph GFa in diesem Intervall streng monoton steigend ist.
2.) Bei 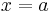 ist der Graph Gfa gleich Null ( Gfa = 0 )und das Steigungsverhalten von GFa ändert für
ist der Graph Gfa gleich Null ( Gfa = 0 )und das Steigungsverhalten von GFa ändert für 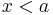 und
und 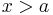 das Vorzeichen. Deshalb kann man sagen, dass der Graph GFa an der Stelle
das Vorzeichen. Deshalb kann man sagen, dass der Graph GFa an der Stelle  einen Extrempunkt, in diesem Fall einen Tiefpunkt ( Minimum ) hat, da sich das Monotonieverhalten von streng monoton fallend nach streng monoton steigend ändert.
einen Extrempunkt, in diesem Fall einen Tiefpunkt ( Minimum ) hat, da sich das Monotonieverhalten von streng monoton fallend nach streng monoton steigend ändert.
2. Bestimmung einer Stammfunktion von fa durch partielle Integration
Hilfe zur partiellen Integration
![\int_a^b f(x)\cdot g'(x)\,\mathrm{d}x
= [f(x)\cdot g(x)]_{a}^{b} - \int_a^b f'(x)\cdot g(x)\,\mathrm{d}x.](/images/math/1/1/e/11e7c88703f100ef6b6c1417e08344b0.png)
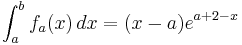
Definiere:

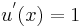
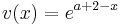
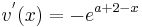
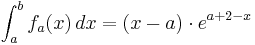
für Interessierte: Der Holzweg
3. Flächenberechnung, der sich nach rechts ins Unendliche erstreckenden Fläche, zwischen der x- Achse und der Funktion f2 im I. Quadranten
- Hinweis:
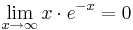
- Hinweis:
Da die Nullstelle der Funktion fa bei x = a liegt, folgt daraus, dass die Nullstelle der Funktion f2 bei x = 2 liegt. Das heißt, man muss von zwei bis unendlich integrieren.
![\int_{2}^{b} f_a( x ) = [-e^{2 + 2 - x}\cdot ( x - 2 + 1 )]^{b}_{2}](/images/math/2/b/6/2b6a89c162d9bf0b6afc66cd92b55004.png)
Der Flächeninhalt, der sich nach rechts ins Unendliche erstreckt, beträgt e2.
![=[( x - a )\cdot (-e^{a + 2 - x}) ]^{b}_{a} - \int_{a}^{b} 1 \cdot (-e^{a + 2 - x})\,dx](/images/math/4/8/b/48b076ebcbea4a660fecb98b42bdd1b2.png)
![=[( x - a )\cdot (-e^{a + 2 - x}) - e^{a + 2 - x}]^{b}_{a}](/images/math/d/4/c/d4c95a12232d92ce7665b2c4e3bc265a.png)
![=[-e^{a + 2 - x}\cdot ( x - a + 1 )]^{b}_{a}](/images/math/a/f/2/af2389785425e5739ed3a370b7ca5ae7.png)
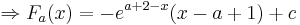
![= [-e^{4 - x}\cdot ( x - 1 )]^{b}_{2}](/images/math/4/f/4/4f4d485b63c946ec1a72aecae0910da1.png)
![= \lim_{b\to\infty} [-e^{4 - b}\cdot ( b - 1 )] - [-e^{4 - 2}\cdot ( 2 - 1 )]](/images/math/e/c/0/ec0cb3d975663bf33b077610026f62b2.png)
![= \lim_{b\to\infty} [-e^{4 - b}\cdot ( b - 1 )] - [-e^{2}\cdot ( 1 )]](/images/math/2/7/b/27b1eff770381056da4fdad7c27fb86f.png)
![| \lim_{b\to\infty} [-e^{4 - b}\cdot ( b - 1 )] \rightarrow 0| siehe Hinweis](/images/math/5/8/c/58c90c4ca0c3da5143fd54578530a462.png)
![= 0 - [-e^{2}\cdot ( 1 )]](/images/math/3/3/c/33c09d0587afaad1a103e98c1c3d6dc5.png)
![= \, [e^{2}] \approx 7.39](/images/math/a/2/9/a299b481180a6e40600355e851cf25a1.png)


