Potenzen: Unterschied zwischen den Versionen

Aus RMG-Wiki
Wechseln zu: Navigation, Suche
(→‎Die Schachbrettaufgabe)
(-rest)
 
(68 dazwischenliegende Versionen von 10 Benutzern werden nicht angezeigt)
Zeile 1: Zeile 1:
−
==Die Schachbrettaufgabe==
+
__NOTOC__
−
; <font color="orange">Aufgabe zu Potenzen</font>
+
<div style="margin:0; margin-right:4px; margin-left:0px; border:2px solid #f4f0e4; padding: 0em 0em 0em 1em; background-color:#f4f0e4;">
 +
[[Jahr der Mathematik/Gedichte|'''Gedichte''']]  '''·''' [[Jahr der Mathematik/Geschichten|'''Geschichten''']]  '''·''' [[Jahr der Mathematik/besondere Zahlen|'''Besondere Zahlen''']] '''·''' [[Jahr der Mathematik/Zahlen im Alltag|'''Zahlen im Alltag''']] '''·''' [[Jahr der Mathematik/Potenzen|'''Potenzen''']] '''·''' [[Jahr der Mathematik/Rap|'''Mathe-Rap''']] '''·''' [[Jahr der Mathematik/Kalender|'''Kalender''']] '''·'''  [[Jahr der Mathematik/Geometrie|'''Geometrie''']] '''·''' [[Jahr der Mathematik/Symmetrie|'''Symmetrie''']] '''·'''  [[Jahr der Mathematik/Quiz und Co|'''Quiz und Co. ''']] 
 +
</div>
 +
==Was sind Potenzen==
  
−
''<font color="dark brown">(Lösung durch Markieren des grauen Feldes sichtbar machen!)</font>''
+
Wenn man ein Produkt aus lauter gleichen Faktoren hat, kann man es kurz als Potenz schreiben. Eine '''Potenz''' besteht aus der '''Basis''' und dem '''Exponent'''. Der Exponent gibt an, wie oft die Basis mit sich malgenommen wird.
  
 +
;Beispiel:
  
−
''<font color="grey">'''Wenn auf dem ersten Feld ein 1 Cent Stück und es sich immer verdoppelt.'''</font>''
+
::::<big>4 <math>\cdot </math>4 <math>\cdot </math> 4  <math>\cdot </math> 4 <math>\cdot </math>  4 = 4<sup>5</sup></big>  
  
−
'''Frage a): '''Wie viele 1 Cent Stücke liegen auf dem 25. Feld?
+
::::<big>Die Basis ist 4 und der Exponent ist 5.</big>
−
     
+
−
'''Frage b): '''Wie viele Euro und Cent Stücke liegen auf allen Feldern?
+
  
−
'''Frage c): '''Wenn man die 1 Cent Stücke vom 25. Feld stabelt und jedes 1,7mm dick ist. Wie hoch ist der Stapel?
+
==Die Schachbrettaufgabe 1==
 +
;Auf dem ersten Feld liegt ein 1 Cent Stück, auf dem zweiten doppelt so viel, auf dem dritten wieder das Doppelte.
 +
'''Berechne oder schätze:'''
 +
''<font color="orange">(Lösung durch Markieren des grauen Feldes sichtbar machen!)</font>''
  
−
'''Frage d): '''Wenn ein 1 Cent Stück 2,3g wiegt, wie viele Tonnen, Kilogramm, Gramm wiegen die 1 Cent Stücke auf dem 25. Feld?
+
#Wie viele 1 Cent Stücke liegen auf dem 2-ten, 3-ten, 4-ten,...25-ten Feld? ''Schau in die Tabelle!''
−
 
+
#Wie viele Euros liegen auf allen Feldern? <u style="color:lightgrey;background:lightgrey"> 335544,33Euro </u>
−
 
+
#Wenn man die 1 Cent Stücke vom 25. Feld stabelt und jedes 1,7mm dick ist. Wie hoch ist der Stapel? <u style="color:lightgrey;background:lightgrey"> 28km,521m,26cm,7mm </u>
−
 
+
#Wenn ein 1 Cent Stück 2,3g wiegt, wie viele Tonnen, Kilogramm, Gramm wiegen die 1 Cent Stücke auf dem 25. Feld? <u style="color:lightgrey;background:lightgrey"> 38t,587kg,596g </u>
−
 
+
 
−
;Lösungen
+
::''(ist vergleichbar mit einem Kampfpanzer)''
−
 
+
−
 
+
−
 
+
−
'''Lösung a)'''=Tabelle gantz rechtes unteres Ergebnis
+
−
 
+
−
'''Lösung b)'''<u style="color:#F34F55;background:#F34F55"> 335544,33Euro </u>
+
−
     
+
−
'''Lösung c)'''<u style="color:#F34F55;background:#F34F55"> 28km,521m,26cm,7mm </u>
+
−
 
+
−
'''Lösung d)'''<u style="color:#F34F55;background:#F34F55"> 38t,587kg,596g </u>
+
−
 
+
−
''(ist verkleichbar mit einem Kampfpanzer)''
+
  
  
Zeile 43: Zeile 36:
 
<center><u style="color:lightgrey;background:lightgrey">8 Cent</u></center>
 
<center><u style="color:lightgrey;background:lightgrey">8 Cent</u></center>
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
−
<center><u style="color:lightgrey;background:lightgrey"> 16Cent </u></center>
+
<center><u style="color:lightgrey;background:lightgrey"> 16 Cent </u></center>
 
|-
 
|-
  
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
−
<center><u style="color:lightgrey;background:lightgrey"> 32Cent </u></center>                                             
+
<center><u style="color:lightgrey;background:lightgrey"> 32 Cent </u></center>                                             
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
−
<center><u style="color:lightgrey;background:lightgrey"> 64Cent </u></center>
+
<center><u style="color:lightgrey;background:lightgrey"> 64 Cent </u></center>
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
−
<center><u style="color:lightgrey;background:lightgrey"> 128Cent </u></center>
+
<center><u style="color:lightgrey;background:lightgrey"> 128 Cent </u></center>
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
−
<center><u style="color:lightgrey;background:lightgrey"> 256Cent </u></center>
+
<center><u style="color:lightgrey;background:lightgrey"> 256 Cent </u></center>
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
−
<center><u style="color:lightgrey;background:lightgrey"> 512Cent </u></center>
+
<center><u style="color:lightgrey;background:lightgrey"> 512 Cent </u></center>
 
|-
 
|-
  
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
−
<center><u style="color:lightgrey;background:lightgrey"> 1024Cent </u></center>
+
<center><u style="color:lightgrey;background:lightgrey"> 1024 Cent </u></center>
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
−
<center><u style="color:lightgrey;background:lightgrey"> 2048Cent </u></center>
+
<center><u style="color:lightgrey;background:lightgrey"> 2048 Cent </u></center>
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
−
<center><u style="color:lightgrey;background:lightgrey"> 4096Cent </u></center>
+
<center><u style="color:lightgrey;background:lightgrey"> 4096 Cent </u></center>
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
−
<center><u style="color:lightgrey;background:lightgrey"> 8192Cent </u></center>
+
<center><u style="color:lightgrey;background:lightgrey"> 8192 Cent </u></center>
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
−
<center><u style="color:lightgrey;background:lightgrey"> 16384Cent </u></center>
+
<center><u style="color:lightgrey;background:lightgrey"> 16384 Cent </u></center>
  
 
|-
 
|-
  
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
−
<center><u style="color:lightgrey;background:lightgrey"> 32768Cent </u></center>
+
<center><u style="color:lightgrey;background:lightgrey"> 32768 Cent </u></center>
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
−
<center><u style="color:lightgrey;background:lightgrey"> 65536Cent </u></center>
+
<center><u style="color:lightgrey;background:lightgrey"> 65536 Cent </u></center>
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
−
<center><u style="color:lightgrey;background:lightgrey"> 131072Cent </u></center>
+
<center><u style="color:lightgrey;background:lightgrey"> 131072 Cent </u></center>
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
−
<center><u style="color:lightgrey;background:lightgrey"> 262144Cent </u></center>
+
<center><u style="color:lightgrey;background:lightgrey"> 262144 Cent </u></center>
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
−
<center><u style="color:lightgrey;background:lightgrey"> 524288Cent </u></center>
+
<center><u style="color:lightgrey;background:lightgrey"> 524288 Cent </u></center>
 
|-
 
|-
  
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
−
<center><u style="color:lightgrey;background:lightgrey"> 1048576Cent </u></center>
+
<center><u style="color:lightgrey;background:lightgrey"> 1048576 Cent </u></center>
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
−
<center><u style="color:lightgrey;background:lightgrey"> 2097152Cent </u></center>
+
<center><u style="color:lightgrey;background:lightgrey"> 2097152 Cent </u></center>
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
−
<center><u style="color:lightgrey;background:lightgrey"> 4194304Cent </u></center>
+
<center><u style="color:lightgrey;background:lightgrey"> 4194304 Cent </u></center>
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
−
<center><u style="color:lightgrey;background:lightgrey"> 8388608Cent </u></center>
+
<center><u style="color:lightgrey;background:lightgrey"> 8388608 Cent </u></center>
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
 
| width="100px" height="100px" style="background-color:#FFFFFF"|
−
<center><u style="color:lightgrey;background:lightgrey"> 16777216Cent </u></center>
+
<center><u style="color:lightgrey;background:lightgrey"> 16777216 Cent </u></center>
 
|}
 
|}
  
  
 
                              
 
                              
−
;Hilfen zu Lösungen von der Aufgabe
+
;Tricks und Hilfen zu den Lösungen:
  
−
;1.Potenzschreibweise
+
*Ergebnis auf dem 25.Feld: <big>'''2<small><sup>24</sup></small>'''</big>
−
Um das Ergebnis auf dem 25.Feld zu erhalten:'''2<small><sup>24</sup></small>'''
+
  
−
;2. Trick: 
+
*Summe aller Münzen auf dem Brett: <big>'''2<small><sup>25</sup></small> - 1'''</big>
−
Um das Ergbnis von den Münzen auf dem Brett zu erhalten:'''2<small><sup>24</sup></small> * 2 - 1'''
+
  
−
== Die Schachbrettaufgabe 2 ==
+
von [[Benutzer:Christian Kempf|Christian Kempf]] und [[Benutzer:Niklas Meißner|Niklas Meißner]]
−
*;Wie viele Körner braucht man, wenn man auf ein Schachbrett ins erste Feld ein Korn, ins zweite Feld doppelt so viel, usw.... legt?
+
  
−
{| style="background-color:#F4A460; spacing:0em; padding:0em"
+
== Aufgaben ==
−
| width="100px" height="100px" style="background-color:#FFFFFF"|
+
;Schreibe mit Hilfe von Zehnerpotenzen!
−
<center><span style="font-size:12pt;"><u style="color:#F34F55;background:#F34F55">1 <small><sup>2</sup></small></u></span>  </center>
+
610000000 = <u style="color:lightgrey;background:lightgrey"> 61  <math>\cdot </math> 10<sup>7</sup></u>
  
−
| width="100px" height="100px" style="background-color:#FFFFFF"|
+
40000 =     <u style="color:lightgrey;background:lightgrey"> 4  <math>\cdot </math> 10<sup>4</sup></u>
−
<center><span style="font-size:14pt;"><u style="color:#F34F55;background:#F34F55">2</u></span></center>
+
  
−
| width="100px" height="100px" style="background-color:#FFFFFF"|
+
23000000 = <u style="color:lightgrey;background:lightgrey"> 23  <math>\cdot </math> 10<sup>6</sup></u>
−
<center><span style="font-size:14pt;"><u style="color:#F34F55;background:#F34F55">4</u></span></center>
+
  
−
| width="100px" height="100px" style="background-color:#FFFFFF"|
 
−
<center><span style="font-size:14pt;"><u style="color:#F34F55;background:#F34F55">8</u></span></center>
 
  
−
| width="100px" height="100px" style="background-color:#FFFFFF"|
+
Jede '''natürliche''' Zahl( größer als 1) ist entweder eine '''Primzahl''' oder sie lässt sich in ein '''Produkt aus Primzahlen''' zerlegen (faktorisieren). Zu jeder Zahl gehört eine bestimmte '''Primfaktorzerlegung'''.
−
<center><span style="font-size:14pt;"><u style="color:#F34F55;background:#F34F55">16</u></span></center>
+
  
−
|-
 
  
−
| width="100px" height="100px" style="background-color:#FFFFFF"|
+
;Zerlege 720 in Primfaktoren:
−
<center><span style="font-size:14pt;"><u style="color:#F34F55;background:#F34F55">32</u></span></center>
+
<u style="color:lightgrey;background:lightgrey">  <big>720 = 72 <math>\cdot </math>10 = 8 <math>\cdot </math>9 <math>\cdot </math>2 <math>\cdot </math>5= 2 <math>\cdot </math>2 <math>\cdot </math>2 <math>\cdot </math>3 <math>\cdot </math>3 <math>\cdot </math>2<math>\cdot </math>5=</big></u>
 +
/<u style="color:lightgrey;background:lightgrey"> <big>2<sup>4</sup><math>\cdot </math>3<sup>2</sup><math>\cdot </math>5</big></u>
  
−
| width="100px" height="100px" style="background-color:#FFFFFF"|
+
Von [[Benutzer:Miriam Abert|Miriam Albert]], [[Benutzer:Lang Alicia|Alicia Lang]] und [[Benutzer:Katharina Schneider|Katharina Schneider]].
−
<center><span style="font-size:14pt;"><u style="color:#F34F55;background:#F34F55">64</u></span></center>
+
  
−
| width="100px" height="100px" style="background-color:#FFFFFF"|
+
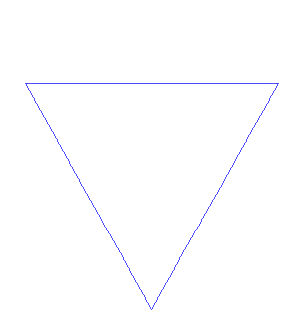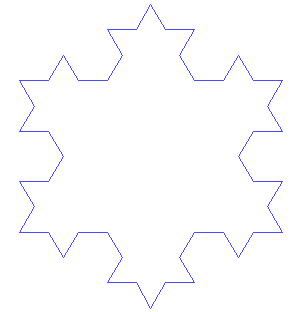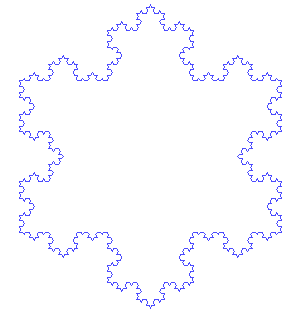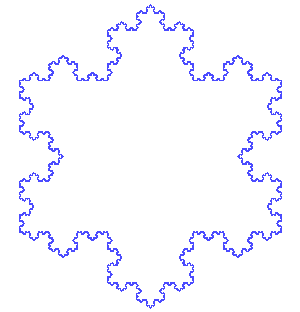
==Schneeflockenkurve==
−
<center><span style="font-size:14pt;"><u style="color:#F34F55;background:#F34F55">128</u></span></center>
+
[[Bild:Von Koch curve.gif]]
  
−
| width="100px" height="100px" style="background-color:#FFFFFF"|
+
;Nun mal langsam!!!               
−
<center><span style="font-size:14pt;"><u style="color:#F34F55;background:#F34F55">256</u></span></center>
+
Die Ausgangsfigur ist        Der '''Stern mit 6 Zacken        Fahre in gleicher Weise fort!
 +
ein Dreieck                  entsteht''', indem man auf
 +
mit gleich langen Seiten:     '''jede Seite''' ein     
 +
                              kleines Dreieck aufsetzt.
 +
                             
 +
[[Bild:Symmetrie Schneeflocke 1.jpg]]      [[Bild:Symmetrie Schneeflocke 2.jpg]]        [[Bild:Symmetrie Schneeflocke 3.jpg]]
  
−
| width="100px" height="100px" style="background-color:#FFFFFF"|
 
−
<center><span style="font-size:14pt;"><u style="color:#F34F55;background:#F34F55">512</u></span></center>
 
  
 +
;Wieviele Seiten besitzt die Schneeflockenkurve?
  
−
|-
+
;1.Schritt: [[Bild:Schritt1.jpg]]
  
−
| width="100px" height="100px" style="background-color:#FFFFFF"|
+
;2.Schritt: [[Bild:Schritt2.jpg]]
−
<center><span style="font-size:14pt;"><u style="color:#F34F55;background:#F34F55">1024</u></span></center>
+
  
−
| width="100px" height="100px" style="background-color:#FFFFFF"|
+
;3.Schritt: [[Bild:Schritt3.jpg]]
−
<center><span style="font-size:14pt;"><u style="color:#F34F55;background:#F34F55">2048</u></span></center>
+
  
−
| width="100px" height="100px" style="background-color:#FFFFFF"|
+
;4.Schritt: [[Bild:Schritt4.jpg]]
−
<center><span style="font-size:14pt;"><u style="color:#F34F55;background:#F34F55">4096</u></span></center>
+
  
−
| width="100px" height="100px" style="background-color:#FFFFFF"|
+
von [[Benutzer:Patrick Jung|Patrick Jung]] nach einer Idee aus dem Schulbuch, Seite 116.
−
<center><span style="font-size:14pt;"><u style="color:#F34F55;background:#F34F55">8192</u></span></center>
+
  
−
| width="100px" height="100px" style="background-color:#FFFFFF"|
 
−
<center><span style="font-size:14pt;"><u style="color:#F34F55;background:#F34F55">16384</u></span></center>
 
  
−
|-
+
== Die Schachbrettaufgabe 2==
−
| width="100px" height="100px" style="background-color:#FFFFFF"|
+
von [[Benutzer:Antje Schramm|Antje Schramm]],[[Benutzer:Anna-Lena Friedrich|Anna-Lena Friedrich]],[[Benutzer: Mara Stahl|Mara Stahl]],[[Benutzer:Luisa Metzner|Luisa Metzner]]
−
<center><span style="font-size:14pt;"><u style="color:#F34F55;background:#F34F55">32768</u></span></center>
+
'''Wie viele Quadrate gibt es auf einem Schachbrett? '''
  
−
| width="100px" height="100px" style="background-color:#FFFFFF"|
 
−
<center><span style="font-size:14pt;"><u style="color:#F34F55;background:#F34F55">65536</u></span></center>
 
  
−
| width="100px" height="100px" style="background-color:#FFFFFF"|
+
Nein, keine 64, auch keine 65 Quadrate.
−
<center><span style="font-size:14pt;"><u style="color:#F34F55;background:#F34F55">131072</u></span></center>
+
  
−
| width="100px" height="100px" style="background-color:#FFFFFF"|
+
Hier eine kleine Hilfe:
−
<center><span style="font-size:14pt;"><u style="color:#F34F55;background:#F34F55">262144</u></span></center>
+
  
−
| width="100px" height="100px" style="background-color:#FFFFFF"|
 
−
<center><span style="font-size:14pt;"><u style="color:#F34F55;background:#F34F55">524288</u></span></center>
 
  
−
|-
 
  
−
| width="100px" height="100px" style="background-color:#FFFFFF"|
+
.....Bild.....    64+?
−
<center><span style="font-size:14pt;"><u style="color:#F34F55;background:#F34F55">1048576</u></span></center>
+
  
−
| width="100px" height="100px" style="background-color:#FFFFFF"|
+
[[Bild:Schachbrett1.jpg]]
−
<center><span style="font-size:14pt;"><u style="color:#F34F55;background:#F34F55">2097152</u></span></center>
+
  
−
| width="100px" height="100px" style="background-color:#FFFFFF"|
 
−
<center><span style="font-size:14pt;"><u style="color:#F34F55;background:#F34F55">4194304</u></span></center>
 
  
−
| width="100px" height="100px" style="background-color:#FFFFFF"|
 
−
<center><span style="font-size:14pt;"><u style="color:#F34F55;background:#F34F55">8388608</u></span></center>
 
  
−
| width="100px" height="100px" style="background-color:#FFFFFF"|
+
'''Lösungsidee'''
−
<center><span style="font-size:14pt;"><u style="color:#F34F55;background:#F34F55">16777216</u></span></center>
+
 
 +
1. Schritt:
 +
 
 +
3x3 Quadrat      3x3 Quadrat            3x3 Quadrat
 +
9 kleine        4 sich überlappende    1 großes
 +
Quadrate        Quadrate              Quadrat
  
−
|-}
+
2. Schritt:
  
−
[[Bild:schachbrett.jpg]]
+
4x4 Quadrat      4x4 Quadrat            4x4 Quadrat
 +
16 kleine        9sich überlappende    4 sich überlappende
 +
Quadrate        Quadrate              Quadrate
 +
 +
Lösung durch Makieren des blauen Feldes sichtbar machen:
  
−
*'''Berechnung der Anzahl der Reiskörner auf dem Schachbrett'''<br>
+
<u style="color:#009ACD;background:#009ACD">8²+7²+6²+5²+4²+3²+2²+1²=</u>
−
**'''Anzahl = 2<small><sup>64</sup></small> - 1 = 18.446.744.073.709.600.000'''<br>
+

Aktuelle Version vom 27. September 2010, 23:35 Uhr

Gedichte · Geschichten · Besondere Zahlen · Zahlen im Alltag · Potenzen · Mathe-Rap · Kalender · Geometrie · Symmetrie · Quiz und Co.

Was sind Potenzen

Wenn man ein Produkt aus lauter gleichen Faktoren hat, kann man es kurz als Potenz schreiben. Eine Potenz besteht aus der Basis und dem Exponent. Der Exponent gibt an, wie oft die Basis mit sich malgenommen wird.

Beispiel
4 \cdot 4 \cdot 4 \cdot 4 \cdot 4 = 45
Die Basis ist 4 und der Exponent ist 5.

Die Schachbrettaufgabe 1

Auf dem ersten Feld liegt ein 1 Cent Stück, auf dem zweiten doppelt so viel, auf dem dritten wieder das Doppelte.

Berechne oder schätze: (Lösung durch Markieren des grauen Feldes sichtbar machen!)

  1. Wie viele 1 Cent Stücke liegen auf dem 2-ten, 3-ten, 4-ten,...25-ten Feld? Schau in die Tabelle!
  2. Wie viele Euros liegen auf allen Feldern? 335544,33Euro
  3. Wenn man die 1 Cent Stücke vom 25. Feld stabelt und jedes 1,7mm dick ist. Wie hoch ist der Stapel? 28km,521m,26cm,7mm
  4. Wenn ein 1 Cent Stück 2,3g wiegt, wie viele Tonnen, Kilogramm, Gramm wiegen die 1 Cent Stücke auf dem 25. Feld? 38t,587kg,596g
(ist vergleichbar mit einem Kampfpanzer)


Centstück 100.jpg

2 Cent
4 Cent
8 Cent
16 Cent
32 Cent
64 Cent
128 Cent
256 Cent
512 Cent
1024 Cent
2048 Cent
4096 Cent
8192 Cent
16384 Cent
32768 Cent
65536 Cent
131072 Cent
262144 Cent
524288 Cent
1048576 Cent
2097152 Cent
4194304 Cent
8388608 Cent
16777216 Cent


Tricks und Hilfen zu den Lösungen
  • Ergebnis auf dem 25.Feld: 224
  • Summe aller Münzen auf dem Brett: 225 - 1

von Christian Kempf und Niklas Meißner

Aufgaben

Schreibe mit Hilfe von Zehnerpotenzen!

610000000 = 61 \cdot 107

40000 = 4 \cdot 104

23000000 = 23 \cdot 106


Jede natürliche Zahl( größer als 1) ist entweder eine Primzahl oder sie lässt sich in ein Produkt aus Primzahlen zerlegen (faktorisieren). Zu jeder Zahl gehört eine bestimmte Primfaktorzerlegung.


Zerlege 720 in Primfaktoren

720 = 72 \cdot 10 = 8 \cdot 9 \cdot 2 \cdot 5= 2 \cdot 2 \cdot 2 \cdot 3 \cdot 3 \cdot 2\cdot 5= / 24\cdot 32\cdot 5

Von Miriam Albert, Alicia Lang und Katharina Schneider.

Schneeflockenkurve

Von Koch curve.gif

Nun mal langsam!!! 
Die Ausgangsfigur ist         Der Stern mit 6 Zacken         Fahre in gleicher Weise fort!
ein Dreieck                   entsteht, indem man auf
mit gleich langen Seiten:     jede Seite ein       
                              kleines Dreieck aufsetzt.
                              
Symmetrie Schneeflocke 1.jpg       Symmetrie Schneeflocke 2.jpg        Symmetrie Schneeflocke 3.jpg


Wieviele Seiten besitzt die Schneeflockenkurve?
1.Schritt
Schritt1.jpg
2.Schritt
Schritt2.jpg
3.Schritt
Schritt3.jpg
4.Schritt
Schritt4.jpg

von Patrick Jung nach einer Idee aus dem Schulbuch, Seite 116.


Die Schachbrettaufgabe 2

von Antje Schramm,Anna-Lena Friedrich,Mara Stahl,Luisa Metzner Wie viele Quadrate gibt es auf einem Schachbrett?


Nein, keine 64, auch keine 65 Quadrate.

Hier eine kleine Hilfe:


.....Bild..... 64+?

Schachbrett1.jpg


Lösungsidee

1. Schritt: 

3x3 Quadrat      3x3 Quadrat            3x3 Quadrat
9 kleine         4 sich überlappende    1 großes
Quadrate         Quadrate               Quadrat

2. Schritt: 

4x4 Quadrat      4x4 Quadrat            4x4 Quadrat
16 kleine        9sich überlappende     4 sich überlappende
Quadrate         Quadrate               Quadrate

Lösung durch Makieren des blauen Feldes sichtbar machen:

8²+7²+6²+5²+4²+3²+2²+1²=
Von „http://rmg.zum.de/index.php?title=Jahr_der_Mathematik/Potenzen&oldid=35817“