Geo: Unterschied zwischen den Versionen
Aus RMG-Wiki
(Die Seite wurde neu angelegt: === Aufgabe 1=== Für welche a, b sind die Vektoren <math>\vec{w}=\begin{pmatrix}1\\2\\a\\\end{pmatrix}</math> ; <math>\vec{u}=\begin{pmatrix}b\\3\\-1\\\end{pmatrix}...) |
|||
| Zeile 1: | Zeile 1: | ||
| − | + | == Hausaufgabe 17.11.2008== | |
| − | === Aufgabe 1=== | + | === Aufgabe 1 (41/3)=== |
Für welche a, b sind die Vektoren <math>\vec{w}=\begin{pmatrix}1\\2\\a\\\end{pmatrix}</math> ; | Für welche a, b sind die Vektoren <math>\vec{w}=\begin{pmatrix}1\\2\\a\\\end{pmatrix}</math> ; | ||
<math>\vec{u}=\begin{pmatrix}b\\3\\-1\\\end{pmatrix}</math> linear abhängig? | <math>\vec{u}=\begin{pmatrix}b\\3\\-1\\\end{pmatrix}</math> linear abhängig? | ||
| + | |||
| + | === Aufgabe 2 (42/4)=== | ||
| + | Zeige, dass die Vektoren <math>\begin{pmatrix}1\\0\\0\\\end{pmatrix}</math>, <math>\begin{pmatrix}1\\1\\0\\\end{pmatrix}</math>, <math>\begin{pmatrix}1\\1\\1\\\end{pmatrix}</math> linear unabhängig sind. | ||
| + | |||
| + | Warum sind die Vektoren <math>\begin{pmatrix}1\\1\\0\\\end{pmatrix}</math>, <math>\begin{pmatrix}1\\1\\1\\\end{pmatrix}</math> linear unabhängig? (Für den Nachweis gibt es drei Möglichkeiten.) | ||
Version vom 17. November 2008, 11:09 Uhr
Hausaufgabe 17.11.2008
Aufgabe 1 (41/3)
Für welche a, b sind die Vektoren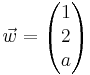 
;
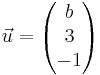linear abhängig?
Aufgabe 2 (42/4)
Zeige, dass die Vektoren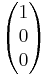 
, 
, 
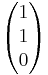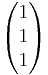linear unabhängig sind.
Warum sind die Vektoren 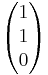, 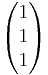linear unabhängig? (Für den Nachweis gibt es drei Möglichkeiten.)
