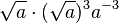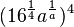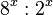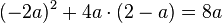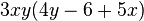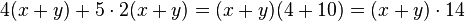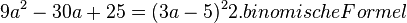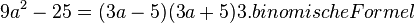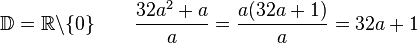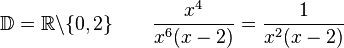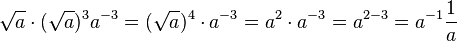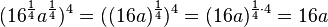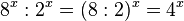Term und Zahl: Unterschied zwischen den Versionen

Aus RMG-Wiki
Wechseln zu: Navigation, Suche
(Die Seite wurde neu angelegt: „test“)
 
Zeile 1: Zeile 1:
−
test
+
== Teste dein Wissen==
 +
 +
 
 +
<br />
 +
<quiz>
 +
{1.Vereinfache folgende Terme soweit wie möglich.
 +
| type="{}" }
 +
<math>\frac48 a-(  \frac24 a- \frac93 b) =</math> { 3b }
 +
<math> -x(-1-x)-(3+x)(4-x)+12=</math> { "0" }
 +
</quiz>
 +
 
 +
 
 +
2.Setze im ersten Term die Klammern so, dass eine richtige Termumformung entsteht. <br />
 +
<math>-2a^2+4a \cdot 2 - a = 8a</math>
 +
<popup name="Lösung">
 +
<math>(-2a)^2+4a \cdot (2 - a) = 8a </math>
 +
</popup> <br />
 +
<math>-2a^3+2b^3+b^3 = -8a^3+4b^3</math>
 +
<popup name="Lösung">
 +
<math>(-2a)^3+2(b^3+b^3) oder -(2a)^3+2(b^3+b^3) = 4a^2+4b^3 </math>
 +
</popup> <br />
 +
 
 +
 
 +
3.Klammere den größtmöglichen Faktor aus.<br/>
 +
<math> 12xy^2-18xy+15x^2y</math>
 +
<popup name = "Lösung">
 +
<math>3xy(4y-6+5x)
 +
</math></popup><br/>
 +
<math> 4(x+y)+5(2x+2y)</math>
 +
<popup name = "Lösung">
 +
<math>4(x+y)+5\cdot2(x+y) = (x+y)(4+10)=(x+y)\cdot14
 +
</math></popup><br/>
 +
 
 +
 
 +
4.Faktorisiere den Term (mit Hilfe der binomischen Formeln)<br/>
 +
<math> 9a^2-30a+25</math>
 +
<popup name = "Lösung">
 +
<math> 9a^2-30a+25=(3a-5)^2 2.binomische Formel
 +
</math></popup><br/>
 +
<math>9a^4-30a^3+25a^2 </math>
 +
<popup name = "Lösung">
 +
<math> 9a^4-30a^3+25a^2=a^2(9a^2-30a+25)=a^2(3a-5)^2 a^2 Ausklammern +2.binomische Formel
 +
</math></popup><br/>
 +
<math>9a^2-25 </math>
 +
<popup name = "Lösung">
 +
<math> 9a^2-25=(3a-5)(3a+5) 3.binomische Formel
 +
</math></popup><br/>
 +
 
 +
 
 +
5. Gib die Definitionsmenge an und vereinfache soweit wie möglich<br/>
 +
<math> \frac{32a^2+a}{a}</math>
 +
<popup name = "Lösung">
 +
<math>\mathbb{D}  =\mathbb{R}  \backslash \{0\}  \qquad \frac{32a^2+a}{a}=\frac{a (32a+1)}{a}=32a+1
 +
</math></popup><br/>
 +
 
 +
<math> \frac{x^4}{x^6(x-2)}</math>
 +
<popup name = "Lösung">
 +
<math>\mathbb{D}  =\mathbb{R}  \backslash \{0,2\} \qquad \frac{x^4}{x^6(x-2)}= \frac{1}{x^2(x-2)}
 +
</math></popup><br/>
 +
 
 +
 
 +
6) Vereinfache soweit wie möglich (a>0) <br/>
 +
<math> \sqrt a \cdot (\sqrt a)^3 a^{-3} </math>
 +
<popup name = "Lösung">
 +
<math> \sqrt a \cdot (\sqrt a)^3 a^{-3} = (\sqrt a)^4 \cdot a^{-3} = a^2 \cdot a^{-3}=a^{2-3}=a^{-1} \frac1 a
 +
</math></popup><br/>
 +
<math> (16^{\frac1 4} a^{\frac1 a})^4</math>
 +
<popup name = "Lösung">
 +
<math>  (16^{\frac1 4} a^{\frac1 4})^4=((16a)^{\frac1 4})^4=(16a)^{\frac1 4 \cdot 4} =16a
 +
</math></popup><br/>
 +
<math> 8^x:2^x</math>
 +
<popup name = "Lösung">
 +
<math>  8^x:2^x=(8:2)^x=4^x
 +
</math></popup><br/>

Version vom 23. Juli 2014, 18:51 Uhr

Teste dein Wissen


Pluspunkt für eine richtige Antwort:  
Minuspunkte für eine falsche Antwort:
Ignoriere den Fragen-Koeffizienten:

1. 1.Vereinfache folgende Terme soweit wie möglich.

\frac48 a-( \frac24 a- \frac93 b) =
-x(-1-x)-(3+x)(4-x)+12=

Punkte: 0 / 0


2.Setze im ersten Term die Klammern so, dass eine richtige Termumformung entsteht.
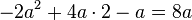
-2a^2+4a \cdot 2 - a = 8a


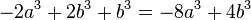
-2a^3+2b^3+b^3 = -8a^3+4b^3



3.Klammere den größtmöglichen Faktor aus.
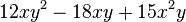
12xy^2-18xy+15x^2y


4(x+y)+5(2x+2y)



4.Faktorisiere den Term (mit Hilfe der binomischen Formeln)
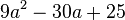
9a^2-30a+25


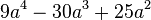
9a^4-30a^3+25a^2


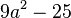
9a^2-25



5. Gib die Definitionsmenge an und vereinfache soweit wie möglich
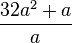
\frac{32a^2+a}{a}


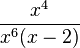
\frac{x^4}{x^6(x-2)}



6) Vereinfache soweit wie möglich (a>0)
\sqrt a \cdot (\sqrt a)^3 a^{-3}


(16^{\frac1 4} a^{\frac1 a})^4


8^x:2^x


Von „http://rmg.zum.de/index.php?title=Mathematik_Grundwissen_10/Term_und_Zahl&oldid=58268“