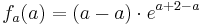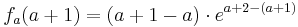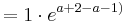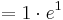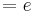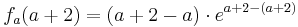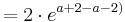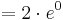Lösung von Teilaufgabe d: Unterschied zwischen den Versionen

Aus RMG-Wiki
Wechseln zu: Navigation, Suche
(→‎Kongruenz der Dreiecke)
Zeile 36: Zeile 36:
 
== Flächeninhalt des Dreiecks ==
 
== Flächeninhalt des Dreiecks ==
  
−
<math>im R^{2}: A = \frac{1}{2}  | ( a_1\cdot  b_2 - a_2\cdot b_1) + (  b_1\cdot c_2 - b_2\cdot c_1 ) + ( c_1\cdot a_2 - c_1\cdot a_2 ) |</math>
+
<math>im R^{2}: A = \frac{1}{2}  | ( a_1\cdot  b_2 - a_2\cdot b_1) + (  b_1\cdot c_2 - b_2\cdot c_1 ) + ( c_1\cdot a_2 - c_1\cdot a_2 ) |</math>
 +
 +
siehe Formelsammlung Seit 81
 +
 
 +
Definiere:
 +
A (a<sub>1</sub> / a<sub>2</sub> ) =  '''R<sub>a</sub>''' ( a / 0 )
 +
B (b<sub>1</sub> / b<sub>2</sub> ) =  '''H<sub>a</sub>''' ( a + 1 / e )
 +
C (c<sub>1</sub> / c<sub>2</sub> ) =  '''W<sub>a</sub>''' ( a + 2 / 2 )
 +
 
 +
<math> A_F = \frac{1}{2}  | ( a\cdot  e - 0\cdot (a+1)) + ( (a+1)\cdot 2 - e\cdot (a+2) ) + ( (a+2)\cdot 0 - 2\cdot a ) |</math>
 +
    <math>  = \frac{1}{2}  |  a\cdot  e - 0 +  2\cdot a + 2- e\cdot a -2\cdot e  +  0 - 2\cdot a ) |</math>
 +
    <math>  = \frac{1}{2}  |  a\cdot  e  +  2\cdot a + 2- e\cdot a -2\cdot e  - 2\cdot a ) |</math>
 +
    <math>  = \frac{1}{2}  |  2 -2\cdot e  |</math>
 +
    <math>  = \frac{1}{2}\cdot2 |  1 - e  |</math>
 +
      <math>  = |  1 - e  |</math>
 +
    <math>\approx  1,718</math>
 +
 +
Der Flächeninhalt beträgt, unabhängig von a, | 1 - e |.

Version vom 5. Januar 2010, 19:17 Uhr

Kongruenz der Dreiecke

Die Dreiecke werden durch die Punkte Ra ( a / f a (a) ), Ha ( a + 1 / f a ( a + 1 )) und Wa ( a + 2 / fa ( a + 2 )) festgelegt.

1.Punkt : Ra ( a / f a (a)) 

     f_a (a) = ( a - a )\cdot e^{ a + 2 - a }

         = 0\cdot e^{ 2 }

         = 0 

   
Der Punkt Ra liegt für alle a bei Ra ( a / 0 )


2.Punkt : Ha ( a + 1 / f a ( a + 1 )) 

    f_a (a+1) = ( a + 1 - a )\cdot e^{ a + 2 - (a+1) }

          = 1 \cdot e^{ a + 2 - a-1) }

          = 1 \cdot  e^{1}

          = e 


Der Punkt Ha liegt für alle a bei Ha ( a + 1 / e )

3.Punkt : Wa ( a + 2 / fa ( a + 2 )) 

    f_a (a+2) = ( a + 2 - a )\cdot e^{ a + 2 - (a+2) }

         = 2 \cdot e^{ a + 2 - a-2) }

         = 2 \cdot  e^{0}

         = 2 


Der Punkt Wa liegt für alle a bei Wa ( a + 2 / 2 )

Mit den nun drei bestimmten Punkten Ra, Ha und Wa lässt sich sagen, dass die Dreiecke kongruent sein müssen. Die y-Werte aller drei Punkte sind für alle a identisch. Daraus schließt man, dass sich die drei Punkte nur auf der x-Achse beziehungsweise auf einer Parallelen zur x-Achse, immer um den gleichen Wert, nämlich um a, verschieben lassen. Da sich die Punkte nur auf Parallelen zur x-Achse verschieben, heißt das natürlich auch, dass sich das Dreieck nur auf der x-Achse verschieben kann und somit immer kongruent ist.

BILD (GEOGEBRA) EINFÜGEN

Flächeninhalt des Dreiecks 

im R^{2}: A = \frac{1}{2}  | ( a_1\cdot  b_2 - a_2\cdot b_1) + (  b_1\cdot c_2 - b_2\cdot c_1 ) + ( c_1\cdot a_2 - c_1\cdot a_2 ) |

siehe Formelsammlung Seit 81

Definiere: 
A (a1 / a2 ) =   Ra ( a / 0 )
B (b1 / b2 ) =   Ha ( a + 1 / e )
C (c1 / c2 ) =   Wa ( a + 2 / 2 )

 A_F = \frac{1}{2}  | ( a\cdot  e - 0\cdot (a+1)) + ( (a+1)\cdot 2 - e\cdot (a+2) ) + ( (a+2)\cdot 0 - 2\cdot a ) |
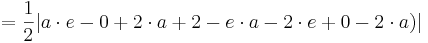
      = \frac{1}{2}  |  a\cdot  e - 0 +  2\cdot a + 2- e\cdot a -2\cdot e  +  0 - 2\cdot a ) |
      = \frac{1}{2}  |  a\cdot  e  +  2\cdot a + 2- e\cdot a -2\cdot e  - 2\cdot a ) |
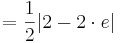
      = \frac{1}{2}  |   2 -2\cdot e   |
      = \frac{1}{2}\cdot2 |   1 - e   |
       = |   1 - e   |
    \approx  1,718

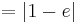
Der Flächeninhalt beträgt, unabhängig von a, | 1 - e |.
Von „http://rmg.zum.de/index.php?title=Facharbeit_Andre_Etzel/Teilaufgabe_d/Lösung_von_Teilaufgabe_d&oldid=25776“